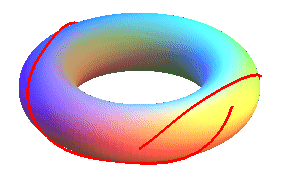
| next curve | previous curve | 2D curves | 3D curves | surfaces | fractals | polyhedra |
|
|
|
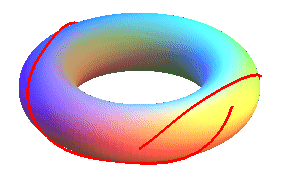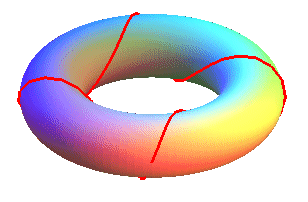
For the torus  :
Differential system of equations:  .
Writing  , differential equation:  , where c is a constant. |
The resolution of the above differential equation leads to elliptic integrals.
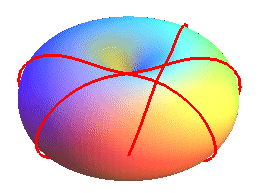
The geodesics can be wound or not; even when they are, they are not toric solenoids (except in the limit cases of meridians and the 2 principal circles).
  |
  |
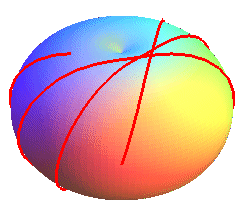| Geodesic of the horn torus | Geodesic of the spindle torus |
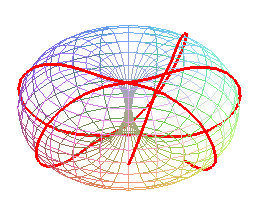
See also the asymptotic lines of the torus.
| next curve | previous curve | 2D curves | 3D curves | surfaces | fractals | polyhedra |
© Robert FERRÉOL 2018