Curve studied by de Moivre in 1718 and Gauss in 1809. Karl Friedrich Gauss (1777 -1855): German astronomer, mathematician, and physicist. Other names: bell curve (of Gauss).

 

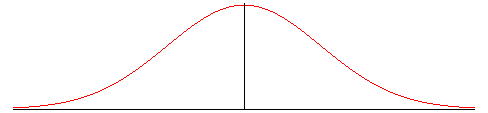
The area between the curve and the asymptote is equal to N; the area of the portion between m - s et m + s is approximately equal to 2/3 of N; between m - 2s and m + 2s it is approximately 96% of N.

Cartesian equation: ,  giving the number of individuals of height between x and x + dx in a "normal" population of N people, with mean height m and a standard deviation s.  For example, the number  of subsets with k elements of a set with n elements can be approximated for large values of n by f(k) with .

The Gaussian curve is the curve of the density function of the normal distribution.

For , we get the Gaussian curve said to be "standard".

Do not mistake the bell curve of the Gaussian distribution with that of the Cauchy distribution, which is none other than a witch of Agnesi.

If we break away from the probabilistic aspect, the Gaussian curve has the following characteristics:
 

Cartesian equation:  ; coordinates of the flex points : . Area between the curve and the asymptote :  ; centroid of this domain : .

© Robert FERRÉOL  2019