


| next curve | previous curve | 2D curves | 3D curves | surfaces | fractals | polyhedra |
LINEAR CELTIC KNOT
| Fantasist page. |
Using the following three basic pieces:
 |
 |
 |
| A, and its left/right flip, that will provide a crossing, | B, and its left/right flip, that will provide two crossings, | M, that will provide three crossings, |
First examples:
| TYPE AA: 1+1 = 2 crossings, Hopf link
|
 |
| TYPE BA: 2+1 = 3 crossings, trefoil knot |
 |
| TYPE BB: 2+2 = 4 crossings, Solomon's knot |
 |
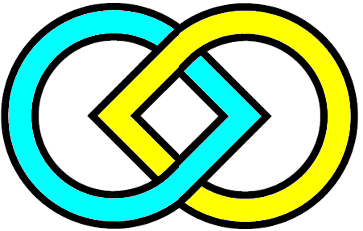
| TYPE AMA: 1+3+1 = 5 crossings, Whitehead link |
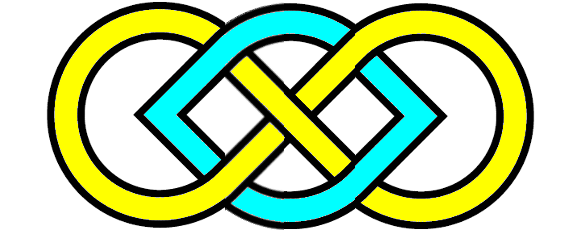 |
| TYPE BMA: 2+3+1 = 6 crossings, knot 6.1.2 |
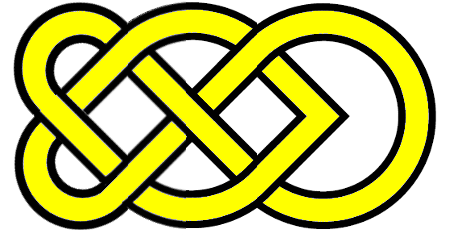 |
| TYPE BMB: 2+3+2 = 7 crossings, knot 7.1.4 |
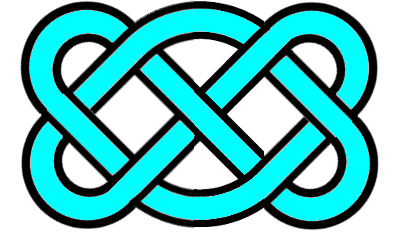 |
| TYPE AM2A: 1+6+1 = 8 crossings, link 8.2.7 |
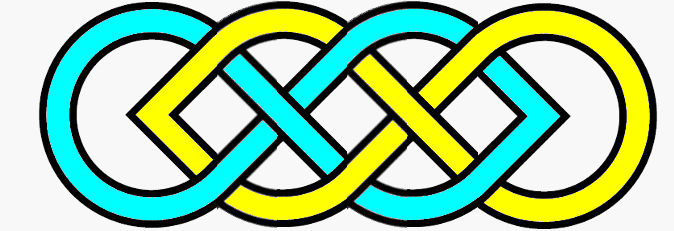 |
| TYPE BM2A: 2+6+1 = 9 crossings, knot 9.1.20 |
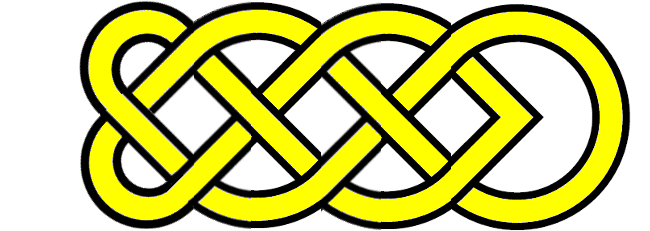 |
| TYPE BM2B: 2+6+2 = 10 crossings, link L10a101 |
 |
Small theorem: the BMnA always are knots, the AMnA always have two blades, and the BMnB, which are rectangular billiard knots of the type (n+2, 2), are knots for odd values of n, and have two blades otherwise.
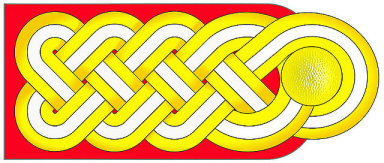
This Wehrmacht officer's epaulette (see here) is a BM3A; knot
K12a541
| next curve | previous curve | 2D curves | 3D curves | surfaces | fractals | polyhedra |
© Robert FERRÉOL 2018