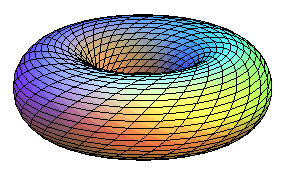
open torus with its rhumb lines
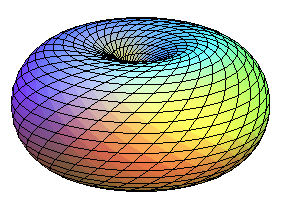
horn torus
| next curve | previous curve | 2D curves | 3D curves | surfaces | fractals | polyhedra |
RHUMB LINE OF THE TORUS

open torus with its rhumb lines |

horn torus |

|
| See this exam paper TPE 96 maths 1 |
| 1st method, in toroidal coordinates.
Differential equation: For the open torus (a > b): The curve is rational (and, in this case, closed) when For the horn torus (a = b): For the spindle torus (a < b) : 2nd method in cylindrical coordinates. Differential equation: For the open torus (a > b): |
| next curve | previous curve | 2D curves | 3D curves | surfaces | fractals | polyhedra |
© Robert FERRÉOL 2018