next surface previous surface 2D curves 3D curves surfaces fractals polyhedra

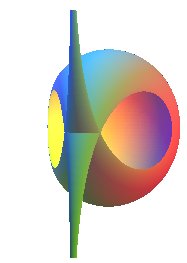
SIEVERT'S SURFACE

Surface studied by Sievert in 1886.
Heinrich Sievert (? - ?): German mathematician.
Websites:
mathworld
An image in glass made by Richard Palais (recognize one of the 5 surfaces).

 
Cylindrical parametrization: .

Sievert's surface is the outcome of the search for a surface with positive constant total curvature, like the sphere, that is not of revolution, and parametrized by usual functions.

See also the surfaces of revolution with constant curvature.
 
next surface previous surface 2D curves 3D curves surfaces fractals polyhedra

© Robert FERRÉOL  2017