Fermat spiral

FERMAT'S SPIRAL

Curve studied by Menelaus in the end of the first century and by Fermat in 1636.
Pierre de Fermat (1601-1655): French mathematician.

Polar equation:

.
Cartesian equation:

.
Transcendental curve.
Curvilinear abscissa:

The Fermat spiral is a special case of parabolic spiral.

It is a closed curve without double points dividing the plane into two connected regions, symmetrical about O.
The blue region opposite corresponds to .

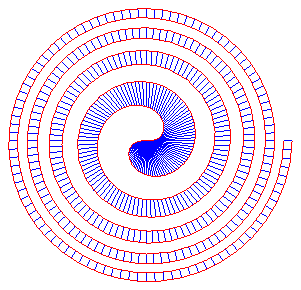If the curve is traced only for nonnegative values of , the area between two consecutive coils is constant equal to .

Its inverse with respect to O is the lituus.

The curve on which it rolls in such a way that the movement of its centre is linear is a cubic parabola.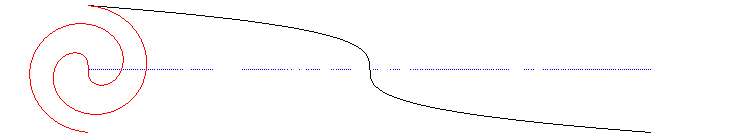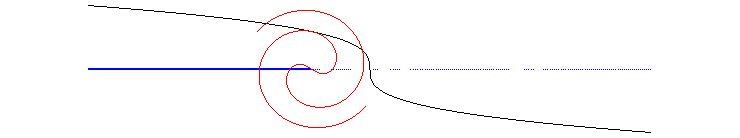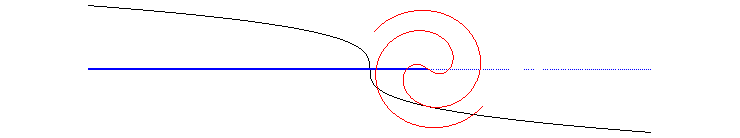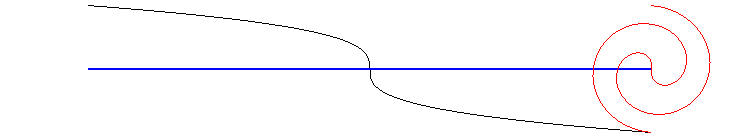

Pre-Columbian work, museum of archaeology, Mexico City.

next curve previous curve 2D curves 3D curves surfaces fractals polyhedra

It is a closed curve without double points dividing the plane into two connected regions, symmetrical about O. The blue region opposite corresponds to .
If the curve is traced only for nonnegative values of , the area between two consecutive coils is constant equal to .