LITUUS
| next curve | previous curve | 2D curves | 3D curves | surfaces | fractals | polyhedra |
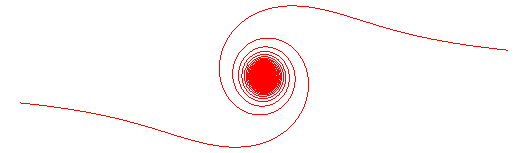
LITUUS

| Curve studied by Cotes and Maclaurin in 1722.
Lituus (Latin word): wand used by Roman augurs, similar to a current bishop's staff. Other name: limaçon spiral. |
 |
| Polar equation: Curvilinear abscissa: Radius of curvature: Transcendental curve. |
 |
The lituus is the locus of the point M on a variable circle centred on O cutting the axis Ox at A such that the area of the circular domain OAM is a constant equal to a2/2.
The inflexion point is obtained for q = 0,5 radians, i.e. around 30°.
The lituus is the inverse with centre O of the Fermat spiral and the radial of the clothoid.
It can be found in the volutes of Ionic capitals:
| next curve | previous curve | 2D curves | 3D curves | surfaces | fractals | polyhedra |
© Robert FERRÉOL 2017