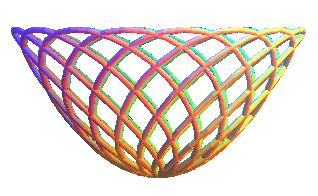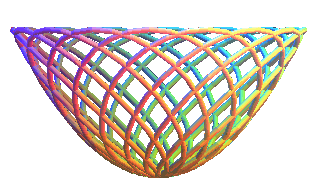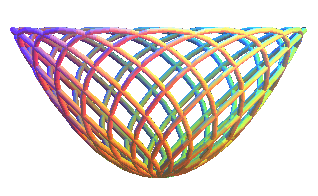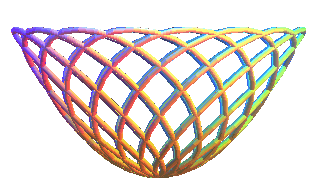
Projection on a plane containing Oz: 2D basin

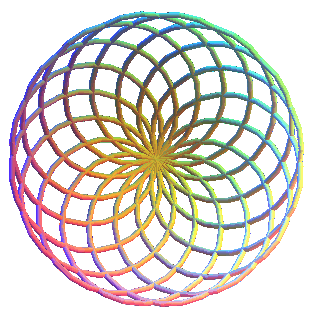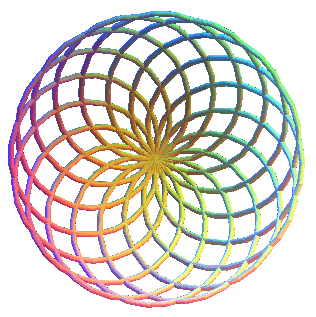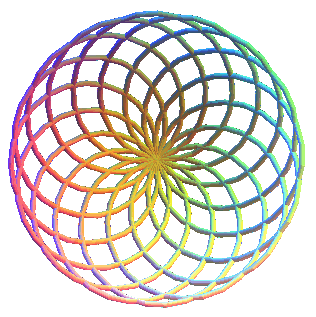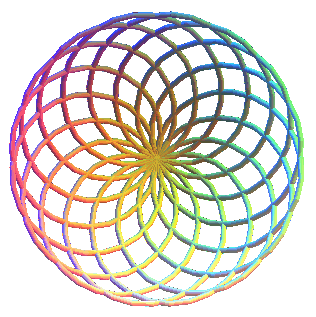
Projection on xOy: rose
| next curve | previous curve | 2D curves | 3D curves | surfaces | fractals | polyhedra |
3D BASIN
|
Projection on a plane containing Oz: 2D basin |
 |

Projection on xOy: rose |
| Homemade name. |
| Cartesian parametrization: Cylindrical equation: |
The basin is the image of the cylindrical sine wave:
by the map
; geometrically, it is therefore the central projection with respect to O of this wave on the paraboloid of revolution:
.
The projections on the planes containing Oz are the 2D basins and the projections on the plane xOy are the roses.
| Animations showing how the 3D basin is the central projection on a paraboloid of a cylindrical sine wave as well as the orthogonal projection of a rose. | 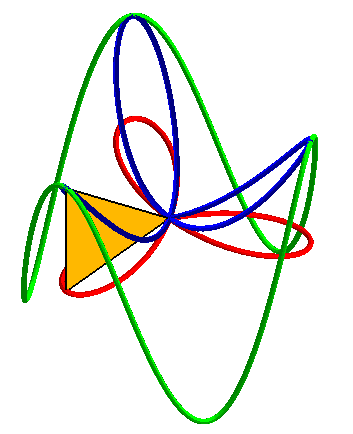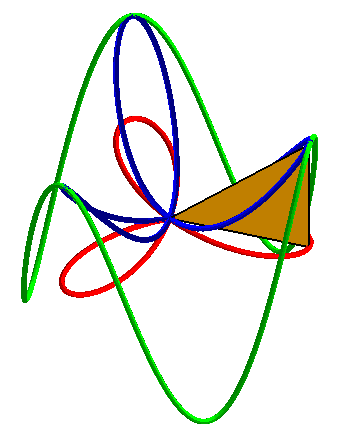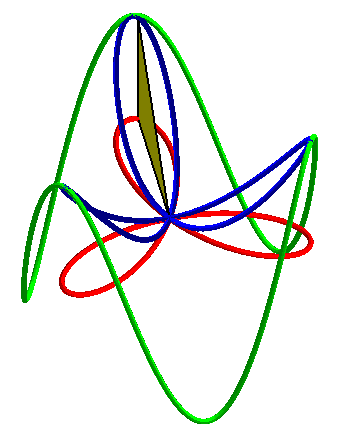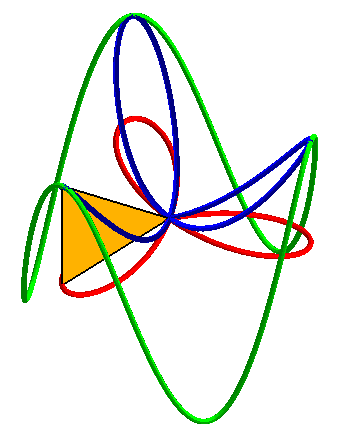
n = 3 |
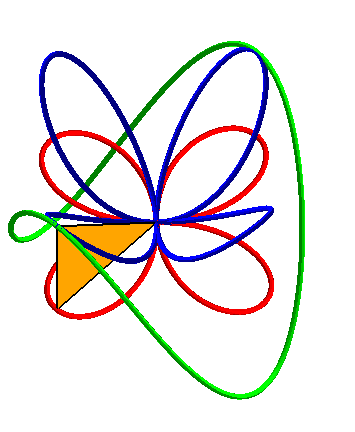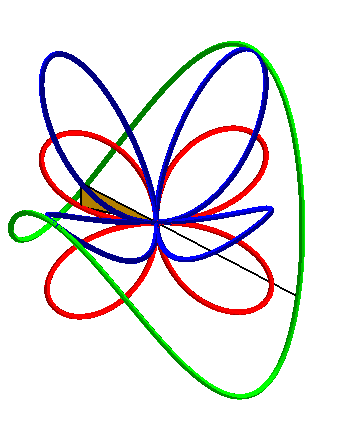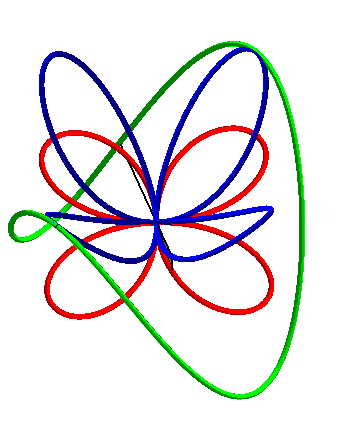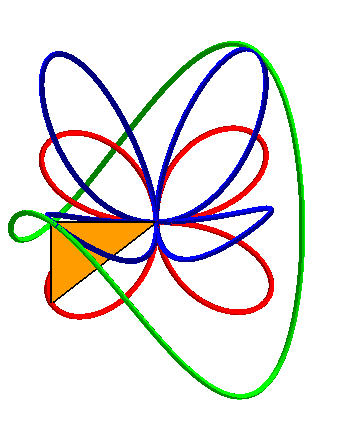
n = 2 |

n = 5/2 |
| Remark: for n =1, the basin |
 |
| In the same way, any curve with polar coordinates Here is, for example, a "lift" of a conchoid of a rose. |
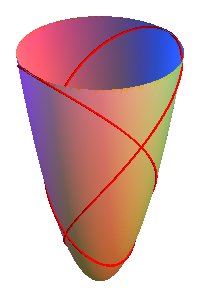 |
Compare to the clelias and the conical roses.
| next curve | previous curve | 2D curves | 3D curves | surfaces | fractals | polyhedra |
© Robert FERRÉOL 2018