Cyclide

CYCLIDE

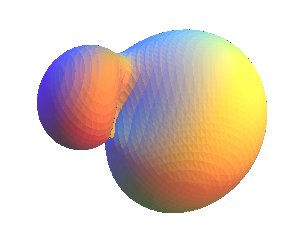
A Darboux cyclide.

From the Greek Kuklos: circle, wheel and eidos: appearance.
Concept studied by Darboux in 1872.
For a historical background, read this thesis on Darboux, page 219.
See also this presentation from 2012.

The cyclides are the envelopes of spheres (C) the centers of which describe a curve or a surface (G₀) (the deferent) and such that a fixed point O has a constant power p with respect to these spheres (this notion is therefore analogous to that of cyclic curve in the plane) ; This notion is equivalent to that of anallagmatic surface.
They are therefore circled surfaces.

The cyclides with a parabola or a paraboloid as a deferent are the spherical cubic surfaces and the cyclides with a conic or a quadric of another kind are the bispherical quartic surfaces, also called "Darboux cyclides".

General equation of the darboux cyclides : .

When the deferent is a conic, the cyclide is called "Dupin cyclide".

next surface previous surface 2D curves 3D curves surfaces fractals polyhedra