HELICO-CONICAL SURFACE
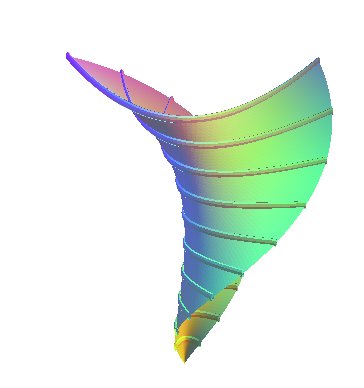
Case of a horizontal parabolic generatrix
| next surface | previous surface | 2D curves | 3D curves | surfaces | fractals | polyhedra |
HELICO-CONICAL SURFACE

Case of a horizontal parabolic generatrix
| Homemade name. Other possibility: helicone. |
| Cartesian parametrization 1: Cartesian parametrization 2: |
The notion of helico-conical surface refers to any surface globally invariant under the action of the set of similarities of the space, products of a rotation around a fixed axis and a homothety centered (at the "vertex") on the axis, with ratio proportional to the angle of rotation.
When the vertex goes to infinity, these surfaces tend to helicoids.
A helico-conical surface is the union of
conical spirals.
Examples:
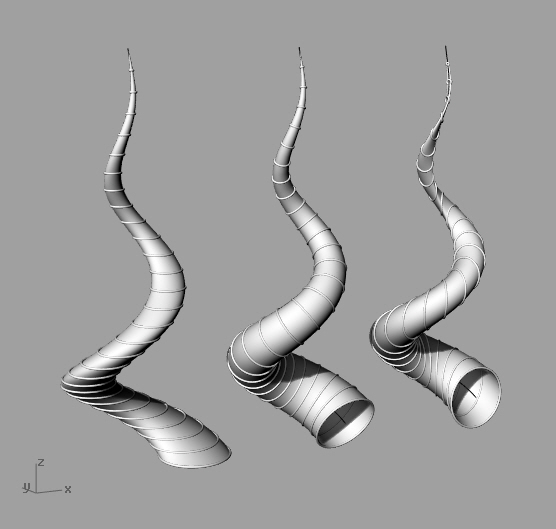
| Case of a circular generatrix.
These surfaces can be referred to by the portmanteau word "helihorns". On the left, horizontal generatrix circle, on the middle, generatrix orthogonal to the central spiral, on the right, vertical generatrix. Compare to the circled helicoids.
|
 |
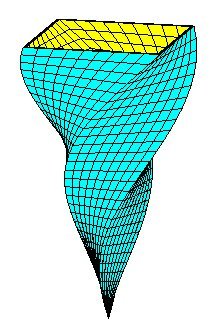
| Case of a linear horizontal generatrix. Assembly of 4 portions of surfaces of this type.
Volume= third of the base times the height, like a pyramid. |
 |
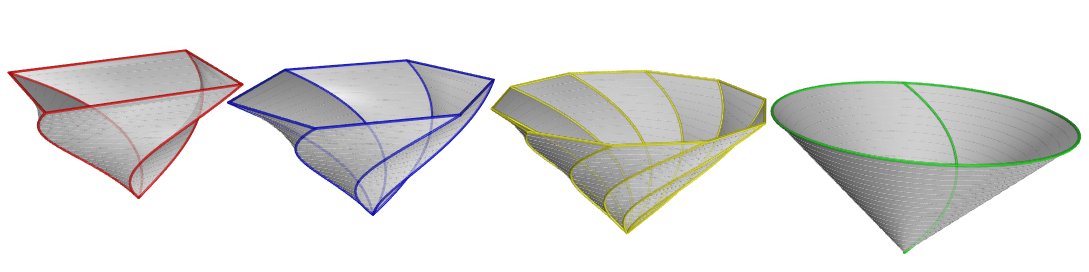
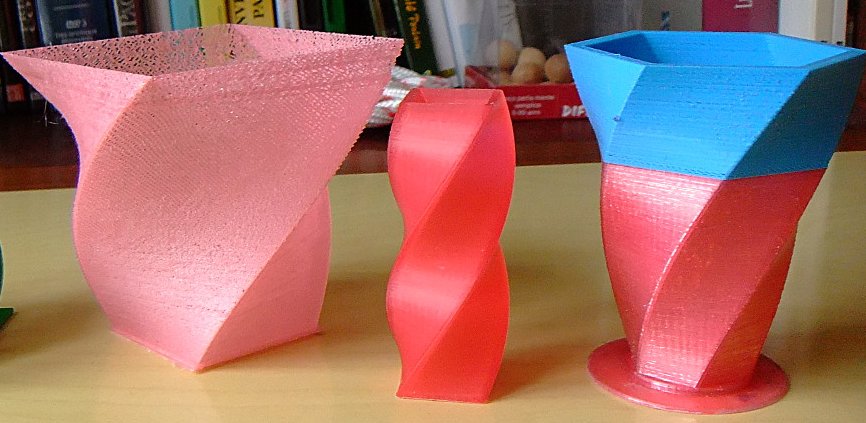
Helico-conical vases, by Alain Esculier.
| next surface | previous surface | 2D curves | 3D curves | surfaces | fractals | polyhedra |
© Robert FERRÉOL 2017