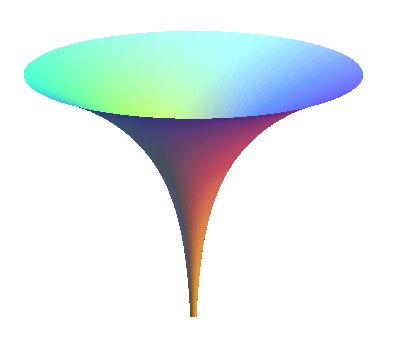
TOWER WITH CONSTANT PRESSURE, OR FUNNEL SURFACE

| next surface | previous surface | 2D curves | 3D curves | surfaces | fractals | polyhedra |
TOWER WITH CONSTANT PRESSURE, OR FUNNEL SURFACE


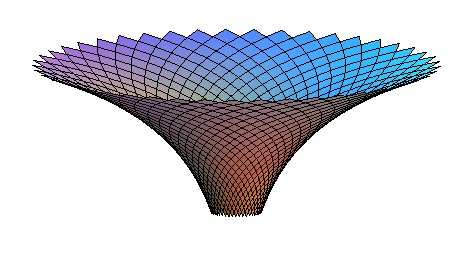
| Cylindrical equation: Cartesian parametrization: First fundamental quadratic form: Second fundamental quadratic form: In the case k = 1, cartesian parametrization the
coordinate lines of which are the asymptotic
lines: |
 |
The tower with constant pressure is the surface
of revolution obtained by rotating a logarithmic
curve around its asymptote.
Its name comes from the fact that, if this surface is
filled with a homogeneous material, then the pressure applied on any horizontal
section by the upper part is constant.
| Derivation of the equation:
The pressure at the altitude z is equal to Remark: if we take the variation of g due to the altitude into account, Much as the first one has a straight line as asymptote, this one has an asymptote cylinder. Note that this tower, extended to infinity, would have an infinite mass, but finite weight... |
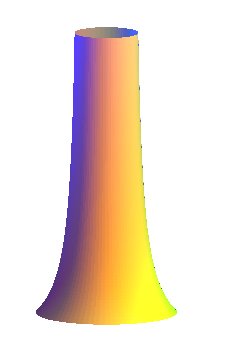 |


See also Gabriel's
horn.
| next surface | previous surface | 2D curves | 3D curves | surfaces | fractals | polyhedra |
© Robert FERRÉOL 2017