CATALAN's CURVE
| next curve | previous curve | 2D curves | 3D curves | surfaces | fractals | polyhedra |
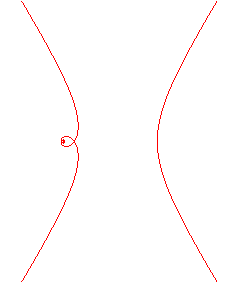
CATALAN's CURVE

| Curve studied by Catalan
in 1856, and by C.
Masurel in 2013.
Eugène Charles Catalan (1814-1894): Franco-Belgian mathematician. |
 |
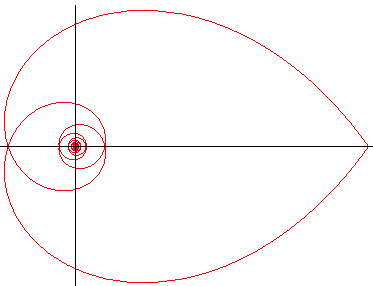 |
Polar equation: Curvilinear abscissa: Curvature radius: |
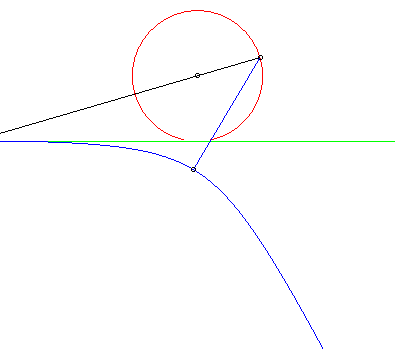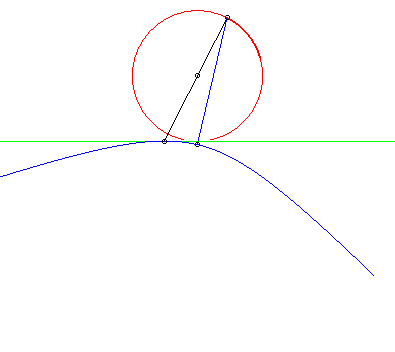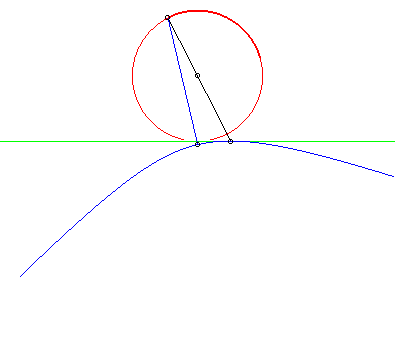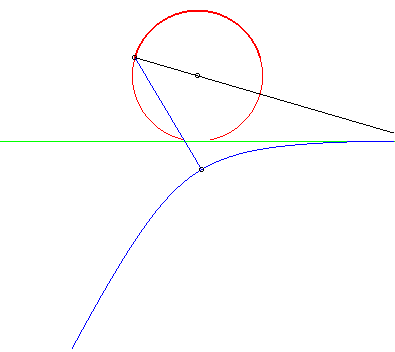 |
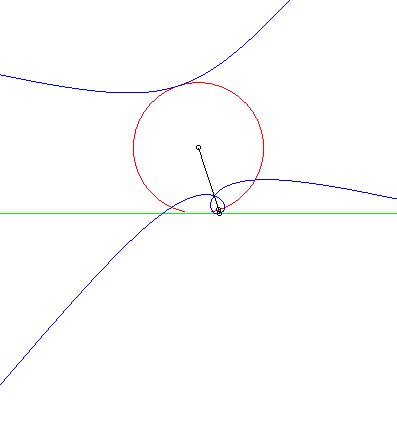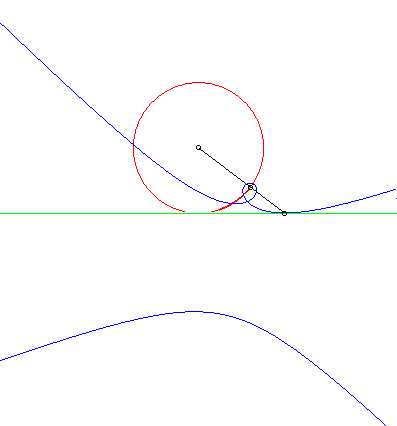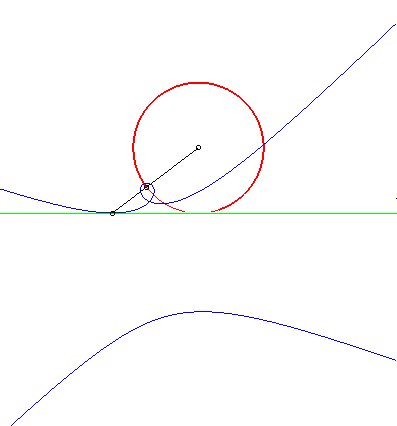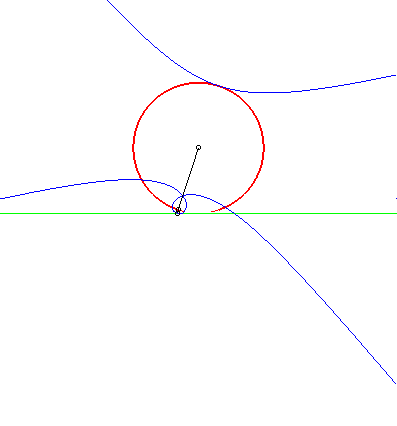
Catalan's curve is the solution of the following problem:
finding the curve that, when rolling without slipping on a line D,
has a point in its plane describing a circle tangent to D.
To put it simply, Catalan's curve is the solution of the problem of the circular roulette. |
 |
As a consequence of the theorem stating that glissettes of a curve, with a linear base, are the roulettes of the evolute of the curve, with a linear base, Catalan's curve is the evolute of the tractrix spiral (which is the solution of the problem of the circular glissette).
Calculating the curvilinear abscissa shows that Catalan's
curve is a solution of the equation ,
whose other solution is the circle.
Writing the polar equation as
shows that it is the polar medial
curve of two hyperbolic spirals.
If we want a point of the plane of the rolling curve to
describe a circle that is not tangent to the base, we get a rolling curve
with polar equation
where a is the radius of the circle and d the distance of
the centre of the circle to the base.
It is a polygasteroid,
evolute of the tractory of a circle.
| next curve | previous curve | 2D curves | 3D curves | surfaces | fractals | polyhedra |
© Robert FERRÉOL
2017