The curves studied on this page are the curves the radius of curvature of which is a sinusoidal function of the curvilinear abscissa.
 

Intrinsic equation 1: . Intrinsic equation 2 when 0  < 1:  Cartesian parametrization:  with ; complex parametrization: . Intrinsic equation 2 when  = 1:  Cartesian parametrization: with ; complex parametrization: . Intrinsic equation 2 when  > 1: . Cartesian parametrization:  with , ; complex parametrization: . Transcendental curve.

 

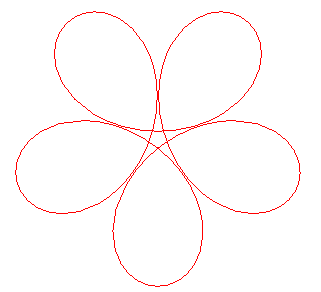
Evolution of a portion of a curve between two points with infinite curvature, when lambda is between 0 and 1.	curve when lambda = 1	curve when n =1 (i.e. lambda = sqrt(2))
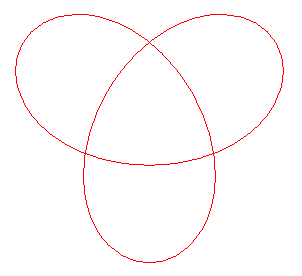
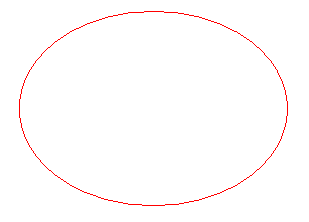
curve when n =3/2 (i.e. lambda = sqrt(13/9))	curve when n =2 (i.e. lambda = sqrt(5))	curve when n =3 (i.e. lambda = sqrt(10))

 
 

If the value of n and lambda are made independent from one another in , we get aesthetically pleasing curves reminding in some cases the hypotrochoids. If n is rational, they are Goursat curves of order the numerator of n. Opposite, n = 5/7, and the values of lambda increase from 1.01.

 

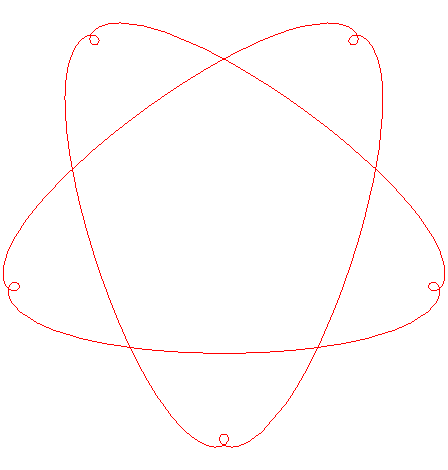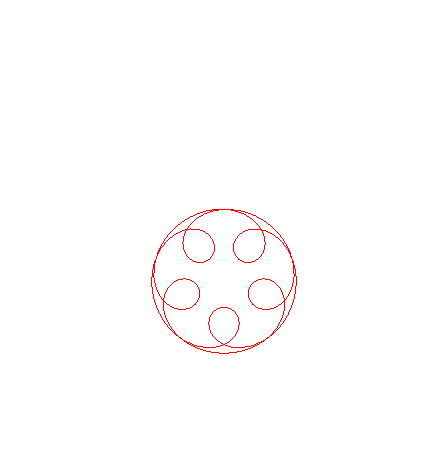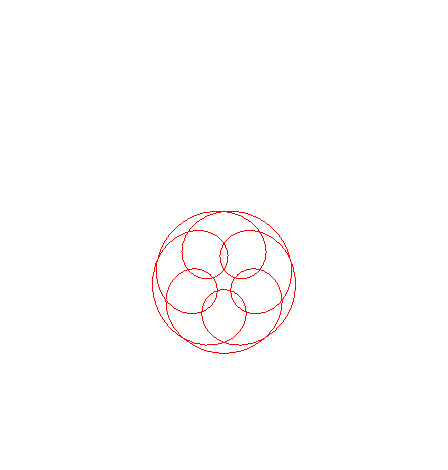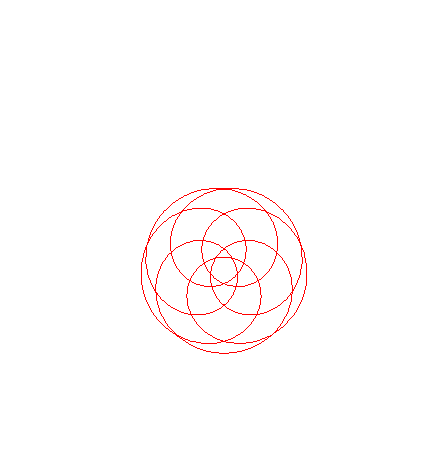If, now, we consider that the amplitude of the sinusoid varies, we can study the family curves with intrinsic equation 1 ,  that are parametrized by  Opposite, an animation with k ranging from 0 to 3, with a stop for k =1 which corresponds the case =0 above.

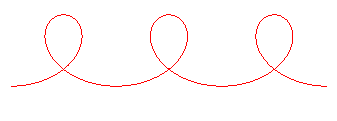
The curves the curvature of which varies as a sinusoidal function of the curvilinear abscissa are the meander curves.

Other curves defined by their intrinsic equation: the clothoid, the curve of constant gyration, the syntractrix curve.

© Robert FERRÉOL 2017