When
Polar equation of the projection on xOy:
| next curve | previous curve | 2D curves | 3D curves | surfaces | fractals | polyhedra |
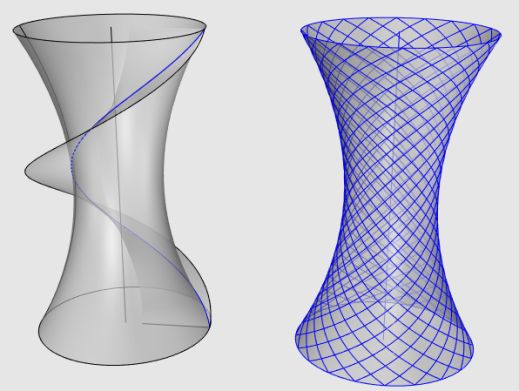
HELIX OF THE ONE-SHEETED HYPERBOLOID OF REVOLUTION
| Curve studied by Blaschke in 1908 [Mh.
Math. Phys. 19, p. 194]
See also [Loria 3d] p. 160. |
| Cartesian parametrization: When Polar equation of the projection on xOy: |
Here, we consider the helix
of the one-sheeted hyperboloid
of revolution with vertical axis, a curve with constant slope
with respect to a horizontal plane.
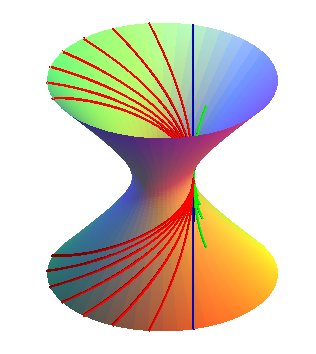
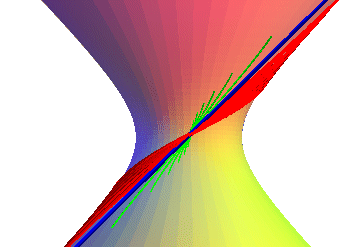
| View of the 3 kinds of helices:
- in red for a slope < b/a, the helix goes to infinity - in blue, the line with slope b/a. - in green for a slope > b/a; the curve has a form of bound (its projection on xOy cannot cross the circle with radius |
 |
 |
Be careful, if the cylindrical helix is indeed the intersection between a right helicoid and a cylinder, this method does not yield the helix of the one-sheeted hyperboloid, but the following curve, that does not have a name:
| Intersection between the helicoid Cartesian parametrization: 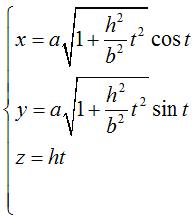 ;
the figure opposite was traced thanks to the parametrization ;
the figure opposite was traced thanks to the parametrization |
 |
| Lift on
the hyperboloid of the spiral |
 |
| View of the construction of these curves (by Robert March). |
 |
Buffon's gloriette in the Jardin des Plantes in Paris...
 |

...made by Alain Esculier. |

Picture: Remy Couderc
| next curve | previous curve | 2D curves | 3D curves | surfaces | fractals | polyhedra |
© Robert FERRÉOL 2018