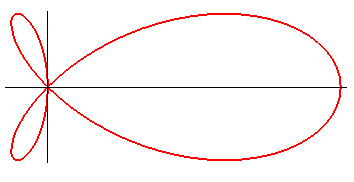
Polar equation: . Cartesian equation: . Cartesian parametrization:  (). Complex parametrization: . Rational quartic. In a frame turned by p/4: Polar equation: . Cartesian equation: .

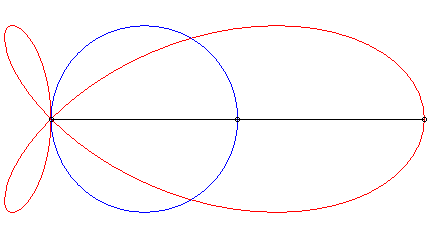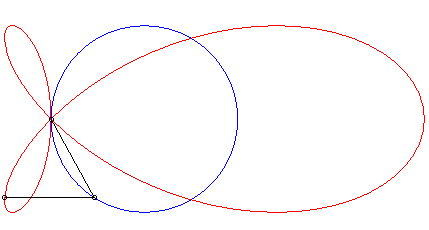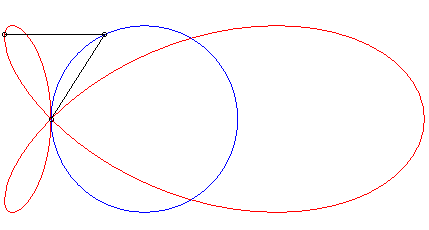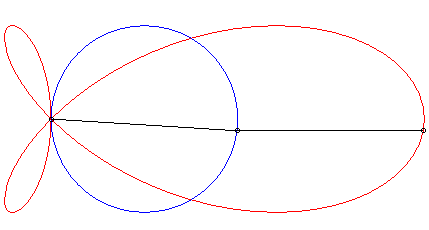
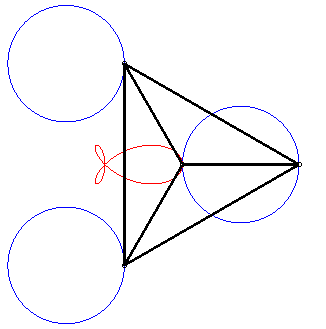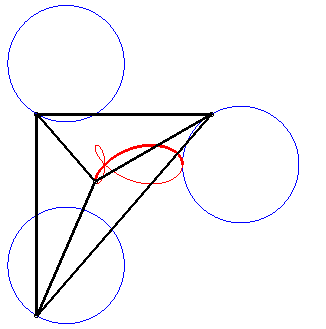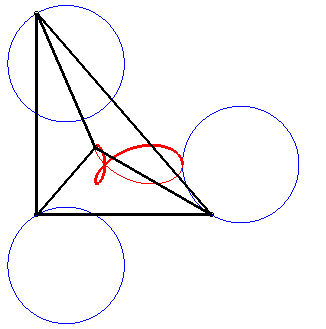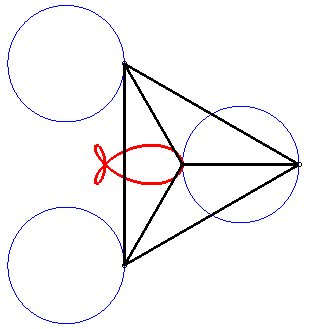
The torpedo is the strophoid of a circle (here the circle with centre (a/4, 0) passing by O) with respect to a point O on the circle and a point A located at infinity in the direction of the diameter passing by O.
It is also a special case of right trifolium (pedal of a deltoid with respect to the point on the inscribed circle that is the symmetric image of the vertex of the deltoid - see folium), of "fish curve" and of tritrochoid (cf the complex parametrization). It is the locus of the isobarycentre of 3 circular motions with same radius, two of which have opposite speeds while the other has double speed.
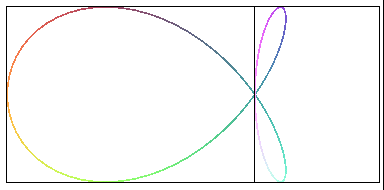
Like all "fish" curves, the torpedo is a planar projection of Viviani's window.

It can also be obtained as the orthopolar of a circle.

See equilateral trefoil for a succinct study of its inverse.

A very close variation of the torpedo is called the "Cramer trifolium":
 

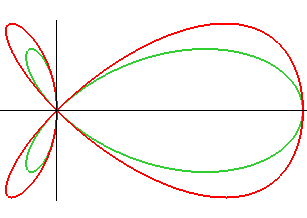Cartesian equation: . Polar equation: . Rational quartics.

Who knows why certain mathematicians studied variations of the torpedo and called them "dickoids":
 

		Tardy dickoid

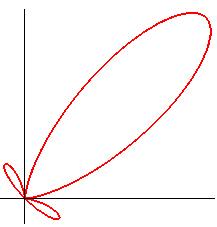next curve

previous curve

2D curves

3D curves

surfaces

fractals

polyhedra

© Robert FERRÉOL  2017