Cuspidal edge

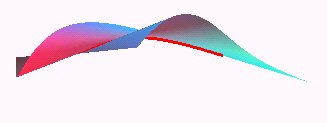
CUSPIDAL EDGE (OR EDGE OF REGRESSION) OF A DEVELOPABLE SURFACE

The generatrix of a ruled developable surface (S) (other than a cone or a cylinder) is always tangent to a curve (G) called cuspidal edge of the surface; the planes tangent to the surface are the osculating planes of the cuspidal edge.

The name cuspidal edge comes from the fact that the section of the surface (S) by a non tangent plane passing by M has a cuspidal point at M.

Example: the cuspidal edge of a developable helicoid is a circular helix.
In fact, any non planar curve is the cuspidal edge of its tangent developable.

When the surface is ruled but not developable, the corresponding notion is that of striction line.

The notion of cuspidal edge can also be generalized to any surface that is the envelope of a family of surfaces with one parameter: the cuspidal edge is then the envelope of the characteristic curves.

next curve previous curve 2D curves 3D curves surfaces fractals polyhedra