
n = 1

n = 2
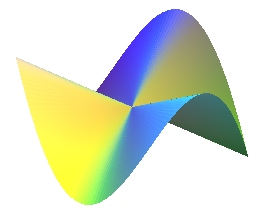
n = 3

n = 4
n =1/2
| next surface | previous surface | 2D curves | 3DÂ curves | surfaces | fractals | polyhedra |
SINUSOIDAL CONE

n = 1 |

n = 2 |

n = 3 |

n = 4 |
|
n =1/2 |
|
| Cylindrical equation: Cartesian parametrization: Algebraic surface when n is rational. |
The section by a cylinder with axis Oz is the reunion of two sine wave cylinders (symmetric images of one another about O).
The section by a sphere centered on O is the reunion
of two cylindric
sine waves (symmetric images of one another about O).
The section by a horizontal plane z = b is an
epispiral: 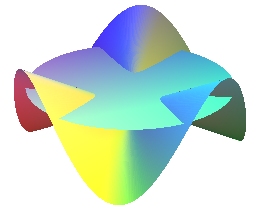 .
.
Compare to Plücker's
conoid.
| We can also consider the secantoidal cone, with
cylindrical equation |
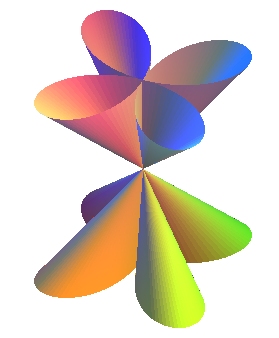 |
Pencil shaving, by Levi Capareda
| next surface | previous surface | 2D curves | 3D curves | surfaces | fractals | polyhedra |
© Robert FERRÉOL 2017