Writing the colatitude, spherical equation: , the axis being Oz and the half-angle at the vertex . Cylindrical equation: . Cartesian equation: . Cartesian parametrization:   (). Parametrization stemming from the polar coordinates  in the plane of development: . First fundamental quadratic form: . Surface element: . Second fundamental quadratic form:   ; . Principal radii of curvature: ; all the points are parabolic. The Cartesian equation of the right cone with directrices Ox and Oy and axis the line y = x, z = 0 is: ; The Cartesian equation of the cone with directrices OxOy and Oz and axis the line x = y = z is: xy + yz + zx = 0 (the angle at the vertex is equal to arccos (-1/3) » 109 ° 28 ') Volume of a trunk of cone with height h and base radius R:  Corresponding area: .

Another parametrization:  (the coordinate lines are Viviani curves).
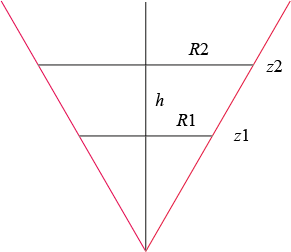
Volume of the trunc of cone limited by the planes  et  : ; see neiloïde.

The cone can be developed by mapping a point M of the cone to the point of the plane with polar coordinates ; a half-cone will then become an angular domain with angle .

Remarkable curves traced on the cone of revolution:
 

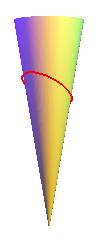
- give honor where honor is due: the planar sections or conics : ellipses, parabolas and hyperbolas. They can be developped into the polygasteroids of index n >1. Cutting by the plane  gives an ellipse, a parabola, or a hyperbola, depending on whether  is greater, equal, or less than ; if the cone rolls over a plane, it is developped into the polygasteroid with polar equation: . Opposite, the case , with the developped polygasteroid.
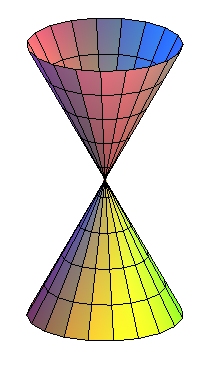
- the curvature lines, which are the parallels (circles) and the meridians (lines)
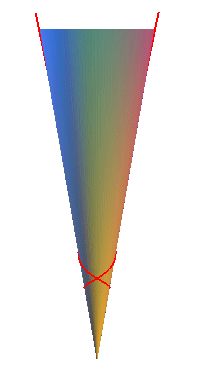
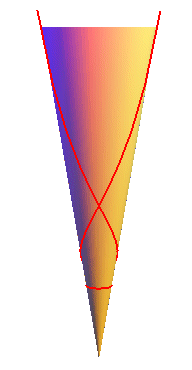
- the geodesics, which are the curves that develop into lines; there are the generatrices and the curves with spherical equation:  , which project on xOy as epispirals with equation:  and develop into the lines .
- the helices, which also are the rhumb lines, which project on xOy as logarithmic spirals: see conical helix.
- the conical spirals of Pappus, that project on xOy as Archimedean spirals.
- the hyperbolic conical spirals.
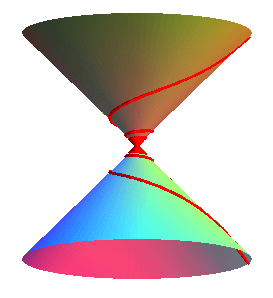- the conical roses, including Viviani's curve.
- the conical catenaries.

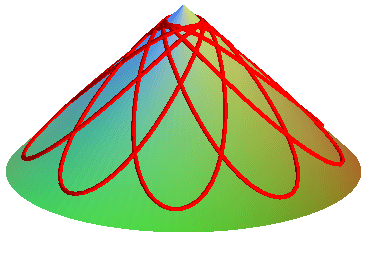See also the spherical cycloids, loci of a point on a cone of revolution rolling without slipping over another cone of revolution, as well as the Cartesian ovals, projections of the intersection between two cones of revolution with parallel axes.

Cones in nature:
 

and elsewhere:
 

next surface

previous surface

2D curves

3D curves

surfaces

fractals

polyhedra

© Robert FERRÉOL 2017