A parabolic conoid is a conoid a directrix of which is a parabola; it is therefore the reunion of the lines supported by a line (D), a parabola (C) and parallel to a plane (P).

When the conoid is right ((D) and (P) perpendicular) and the axis of the parabola is parallel to (D), we get Whitney's umbrella.

Case of the right conoid with axis Oy and directrix the parabola  in the plane x = a :

Cartesian equation : . Ruled cubic surface.

Cas a=b

Cas b= 0

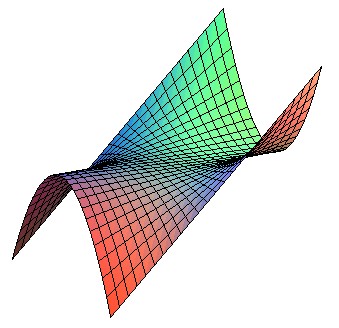This surface is projectively (and really) equivalent to Whitney's umbrella, and therefore to Plücker's conoid .

When the parabola and the axis of the conoid intersect, the surface is a hyperbolic paraboloid.

 

next surface

previous surface

2D curves

3D curves

surfaces

fractals

polyhedra

© Robert FERRÉOL 2020