For surfaces of directrix Oz : Differential cylindrical caracterisation: . Cylindrucal equation: . Cartesian paramétrisation: .

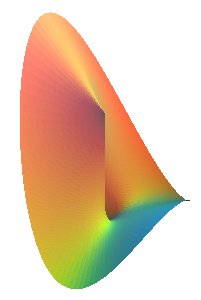
A ruled surface is said to be with straight directrix if its generators all pass through a fixed line.

Examples :
 - cones (case where the directrix is reduced to a point), 
 - conoids (of which the hyperbolic paraboloid), case where there is also a director plane, 
 - conoidal surfaces (of wich the milk cartoon), case where there are two straight directrices
 - closed ruled helicoids (of which the right helicoid), .

See also the ruled surfaces with a director plane.
 

next surface

previous surface

2D curves

3Dcurves

surfaces

fractals

polyhedrons

© Robert FERRÉOL  2020