Case n = 5/16

Case n = 5/7
| courbe suivante | courbe précédente | courbes 2D | courbes 3D | surfaces | fractals | polyèdres |
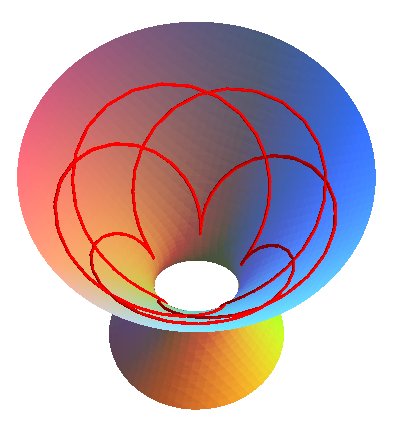
SALKOWSKI CURVE

Case n = 5/16 |

Case n = 5/7 |
| Curve studied by E. Salkowski in 1909, and by J. Monterde in 2009 and in 2024. |
| Cartesian paramétrization : Curve traced on the revolution quadric : Abscisse curviligne : Constant curvature radius : Torsion radius: |
Salkowski curves are curves of constant curvature whose principal normal makes a constant angle with a fixed direction (these latter curves being designated by "slant helices", compare with helices).
For ,
i.e.
,
the curve is plotted on an revolution
ellipsoid, and for
,
i.e.
,
it is plotted on a revolution
hyperboloid.
In the first case, the projection on xOy is the
same as that of the spherical
epicycloid with parameters
and
(see the article
by J. Monterde). The Salkowski curve is therefore formed of arches
whose number is equal to the numerator of 2n if n is rational.
The cuspidal points being obtained for ,
so
, we
get an arch for
,
and the complete curve for
.
| courbe suivante | courbe précédente | courbes 2D | courbes 3D | surfaces | fractals | polyèdres |
© Robert FERRÉOL
2024