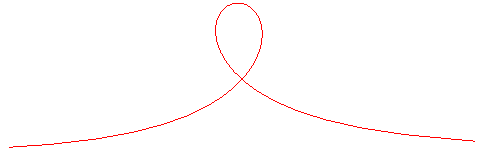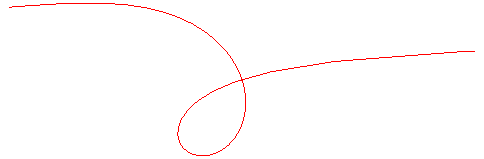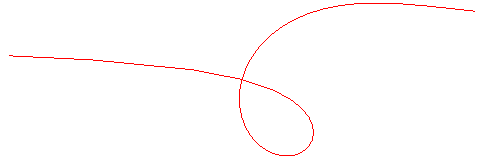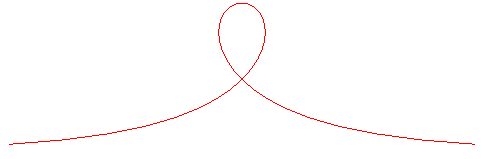
STROPHOID
| next curve | previous curve | 2D curves | 3D curves | surfaces | fractals | polyhedra |
STROPHOID

| Curve studied by Barrow (one of Newton's professors)
in 1669, by Quételet in 1810 and by Chasles; the name was given
by Enrico
Montucci in 1837.
Strophoid comes from the Greek strophos "string, belt, braid". Other name: Quételet focal, nodal focal, harmonic curve. |
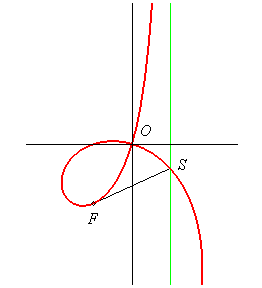 |
Polar equation:
. Focus F Intersection point with the asymptote: S Inflection point obtained for Cartesian equation: Rational circular cubic with a double point. In the frame (F , Cartesian equation: Complex equation: Polar equation: i.e. If |
| Given two points O and F and a line (D0) passing by O, the strophoid with focus F, double point O and axis (D0) is the locus of the points M on a variable line (D) passing by F such that PM = PO, where P is the intersection point of the lines (D) and (D0) (in other words, it is the strophoid of (D0) with respect to O and F). | 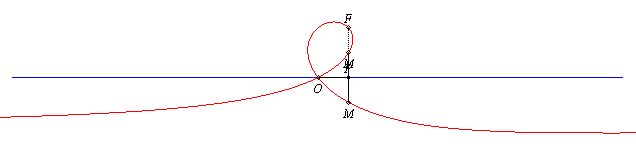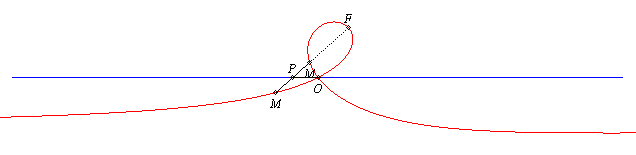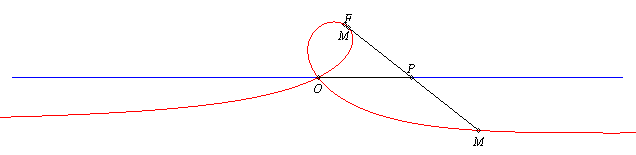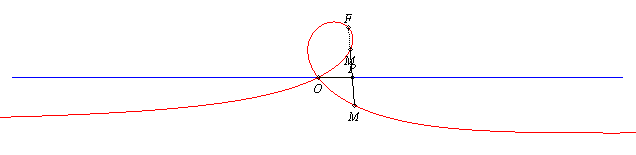
The strophoid is the locus of the points M such that PM = PO |
| The strophoid is therefore also the locus of the contact points of the tangents passing by F to the circles tangent at O to the axis (D0). |
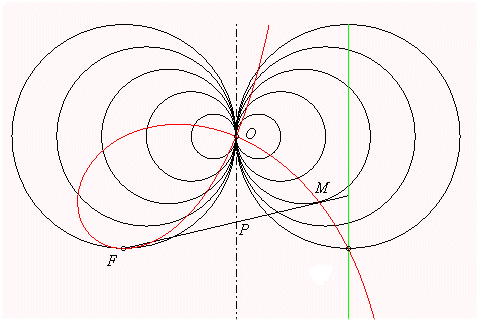 |
It follows from this definition that the axis (D0) of the strophoid is the median with pole O of the strophoid with itself.
Here, O is the origin of the frame, F is
the point ,
and (D0) is the axis Oy;
the asymptote is the line parallel to (D0)
passing by the symmetric image of F about (D0).
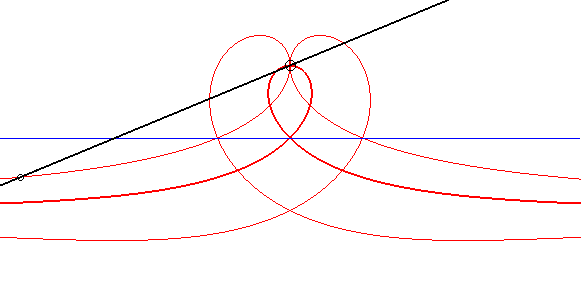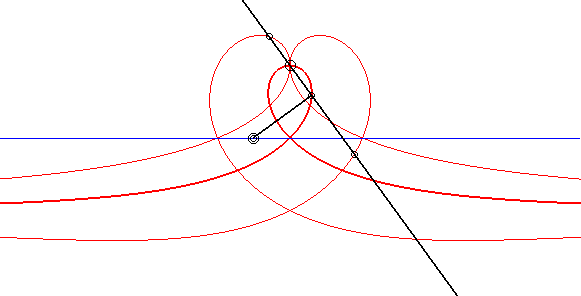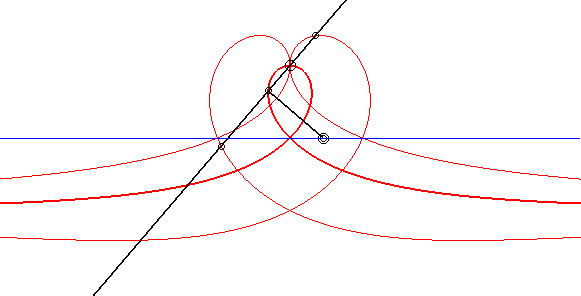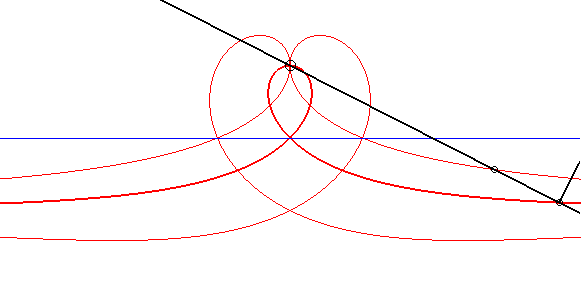
| Animation showing the construction of the right strophoid
by the Newton set-square method, and of two conchoidal strophoids.
See details on the page dedicated to the glissettes. |
 |
The complex equation shows that the strophoids are the
loci of the point M such that, if its image by the composition of
an inversion with centre F and a reflection about (OF) is
called M', then the middle of MM' describes a line (D0)
passing by O; M' is called the conjugate of
M.
 |
The middle I of [M M'] describes the line (D0); M1 is the inverse with pole F of M with respect to the circle (C) and M' is the symmetric image of M1 about (Da ). |
If A and A' are two conjugate points of
the strophoid, M any point on this curve, then the line (OM)
is a bisector of (AMA'); inversely, given 3 unaligned points O,
A, A', the locus of the points
M such that (OM) is a
bisector of (AMA') is a strophoid, and so is the locus of the points
M
"seeing" the oriented segment lines [AO] and [OA'] under
the same angle.
The strophoid is therefore a special case of the isoptic
cubic, locus of the points from where two segment lines can be seen
under the same angle.
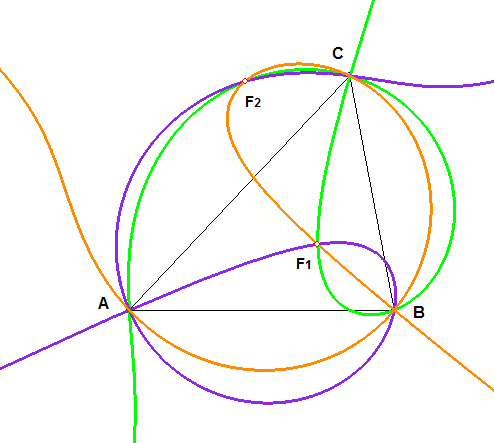
| A non-flat triangle (ABC) thus gives 3 strophoids:
(SA) defined by (MC, MA) = (MA, MB) in mauve (SB) defined by (MA, MB) = (MB, MC) in brown (SC) defined by (MB, MC) = (MC, MA) in green When the triangle is flat, these loci are circles of Apollonius, hence the name strophoids of Apollonius given to these curves. The three curves have, in general, 5 common points: the
3 vertices, the Fermat
point of the triangle F1 , defined
by |
 |
| The strophoid is also found in the following optics problem: an observer B looks at the image A' of a fixed point A in a mirror turning around a fixed point O; the locus of the intersection points of the ray BA' with the mirror is a strophoid with double point O, focus A, and axis parallel to (BE) constructed on the figure. If D is the symmetric image of A about OB, then the line (BD) is the tangent at B. | 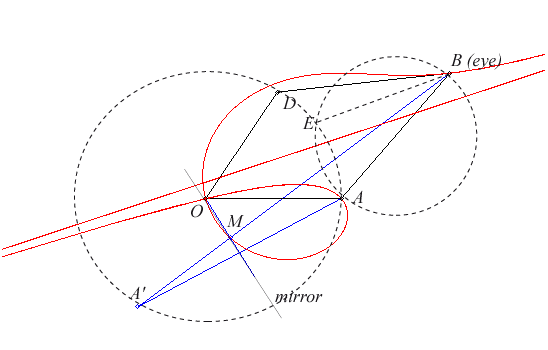 |
The strophoids have another elegant 3D construction discovered
by Quételet, as the locus of the foci of conics (hence the name:
Quételet focal): if S is the intersection point between the
strophoid and its asymptote, and (C) is the cone of revolution with
axis (OS) passing by F (and half-angle at the vertex ),
then the strophoid is the locus of the foci of the conical sections of
the cone (C) by the planes perpendicular to the plane (OSF)
passing by F.
 |
S, vertex of the cone, M and M':
foci of the conic on a plane perpendicular to (OSF) passing by F;
the centre
I of the conic describes the axis of the strophoid.
For the right strophoid, the cone becomes a cylinder. |
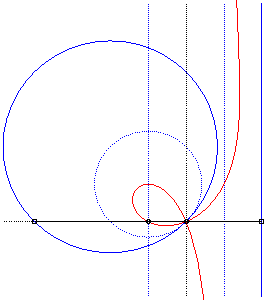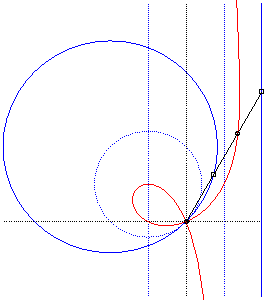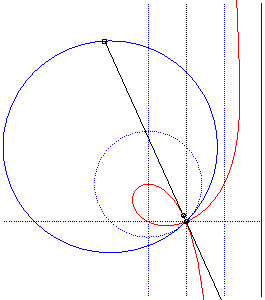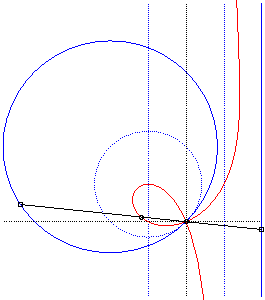
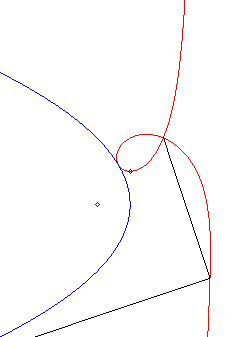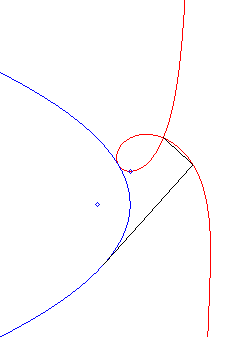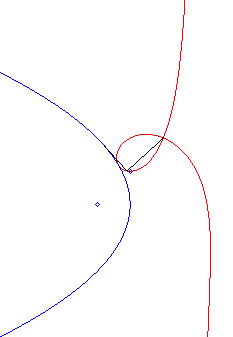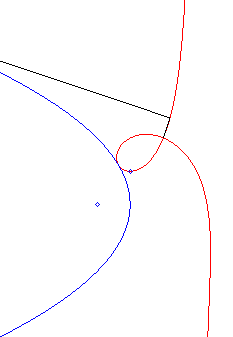
Like all the rational circular cubics, the strophoids can be defined as:
- the cissoids with pole O of a circle passing by O and a line passing by the symmetric image of the centre of the circle about O (here, the circle is the circle (C) with centre F passing by O, the line, the line parallel to (D0) passing by the symmetric image of F about O).
 |
The focus of the strophoid is at the middle of the segment
line joining its double point to the focus of the parabola.
The strophoid is therefore also the envelope of the circles with diameter joining O to a point on the parabola; in other words, it is the cyclic with initial curve, or deferent, a parabola, when the radius of inversion is zero and the pole is on the directrix of the parabola. |
- the inverses of a rectangular hyperbola with respect to one of its points (here O)
See cochleoid
and Mobius surface.
| next curve | previous curve | 2D curves | 3D curves | surfaces | fractals | polyhedra |
© Robert FERRÉOL 2017