Circular helix

CYLINDRICAL HELIX

left-handed helix

right-handed helix

First study (that did not reach our times) credited to Apollonius of Perga (2nd century BC) and second study by Geminus of Rhodes (1st century BC).
Other name: circular helix.

Cartesian parametrization: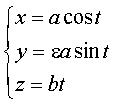

.
System of cylindrical equations:

.
Curvilinear abscissa:

, where

.
Radius of curvature:

, angle of curvature: at / c.
Radius of torsion:

, angle of torsion: bt / c.
Length of a coil:

.
Center of curvature:

(describes another cylindrical helix).

The cylindrical helix can be defined as a helix traced on a vertical cylinder of revolution, or a rhumb line of this cylinder (i.e., in both cases, a curve forming a constant angle with respect to the axis of the cylinder), or a geodesic of this cylinder (in other words, a curve that becomes a line when the cylinder is developed) or a solenoid with linear bore.
Intrinsic characterization: constant curvature and torsion.

The radius of the helix is a, and its shift is (it is the distance between two consecutive coils) and b is sometimes called reduced shift of the helix. The angle of the helix is the constant angle (equal to ) formed by its tangent with respect to any plane orthogonal to Oz. The helix is right-handed when e = 1 (it “goes up” counterclockwise and an observer located outside of it sees it going up from left to right) and left-handed when e = - 1 (it “goes up” clockwise).

The DNA spirals along a double right-handed helix.
The threads of screws or corkscrews are right-handed, as opposed to those of butane bottles. Une glycine gauche Climbing plants wind along helices, either right-handed like for the vine (hence the expression weinwendig in German) or the Chinese wisteria, or left-handed like for the hops (hence the expression hopfenwendig) or the Japanese wisteria (according to Michel the gardener on France-Info radio station).

Finally, in Brazil, a species of bat flying invariably along right-handed helices has been observed.

The trajectories of a charge placed in a uniform magnetic field and subject to the Laplace force is a cylindrical helix with axis parallel to that of the field (or a circle if the velocity is perpendicular to the field, a straight line if it is parallel). Same result for an inelastic flexible wire carrying a continuous electric current placed in the field (and not subject to gravity). The shape assumed by this wire was called by Riebke "electrodynamic catenary", and is in fact a cylindrical helix, with the same limit case as before.

Proof of this fact:
Newton's second law and the expression of the Laplace force give: (1) where is the tension of the wire, the tangent unit vector, the magnetic field and I the intensity of the current;
shows that the norm of the tension is constant: T = constant. (1) can be therefore be written ;
shows that : the tangent forms a constant angle with respect to a fixed direction, the curve is a helix.
The first Frenet formula and (2) yield: the radius of curvature is constant.
The radius of torsion of the helix, classically given by is therefore itself also constant: the curve is a cylindrical helix with axis parallel to the field lines.

Nota: why are the blade-based propelling systems for boats or planes called helices? It is because when the boat moves with constant speed, the motion of the helix with respect to a fixed frame is a helicoidal motion, and all its points describe cylindrical helices.

For the projections of the cylindrical helix, see at trochoid, cochleoid and hyperbolic spiral.
See also the helicoids, the other cylindrical curves, the coil (tube with a cylindrical-helix-shaped bore), and the revolution of the sinusoid.

More beautiful images by Amain Juhel.