KAMPYLE OF EUDOXUS
| next curve | previous curve | 2D curves | 3D curves | surfaces | fractals | polyhedra |
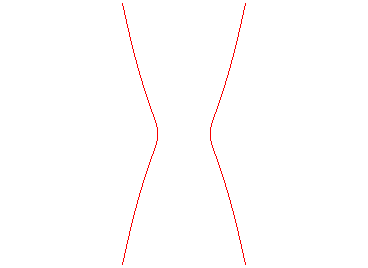
KAMPYLE OF EUDOXUS

| From the Greek Kampulos: curved
Eudoxus of Cnidus (406 BC - 355 BC): Greek astronomer, mathematician and philosopher. Other name: Clairaut's curve. |
 |
Polar equation: Cartesian equation: (compare with that of Gerono's lemniscate). Rational quartic. Equation of the parabolic asymptotes: |
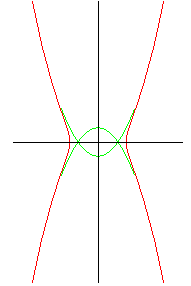
| If for a point P travelling on a circle (C) of centre O, the tangent to C at P cuts Ox in Q, then the Kampyle of Eudoxus is the locus of the intersection point between the line (OP) and the line parallel to Oy passing through Q. |  |
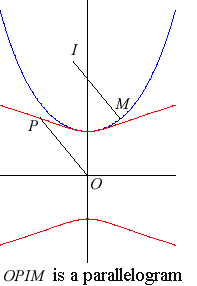
| The Kampyle (in red, opposite) is also the locus of the
focus of a parabola constrained to stay tangent to a straight line at a
fixed point. Therefore, it is a glissette.
For a parabola of parameter p, we get a Kampyle
of parameter a = p/2.
|
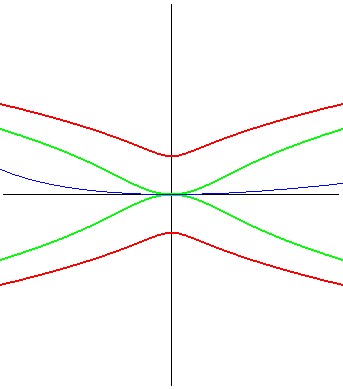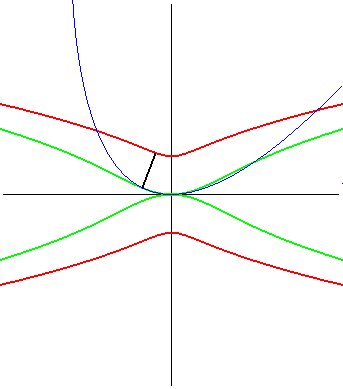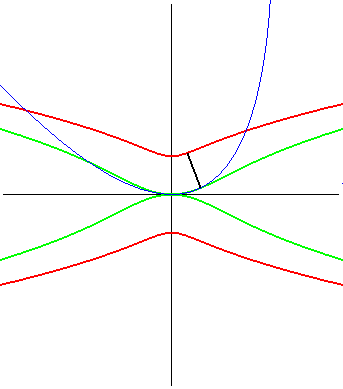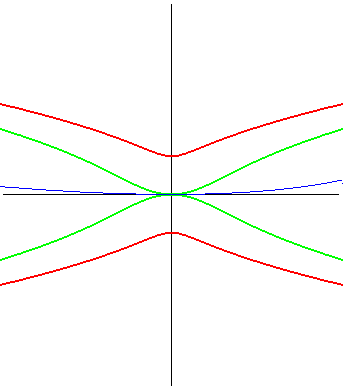 |
The Kampyle of Eudoxus also is the radial curve of the catenary curve (here, up to rotation by an angle of p/2),
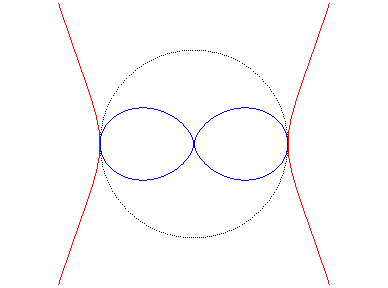
as well as the inverse of the double egg,
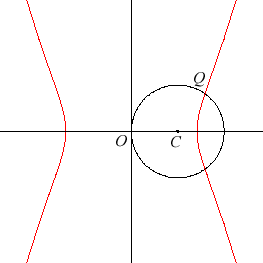
It was studied by Eudoxus because it is a duplicatrix.
Indeed, its intersection point Q with a circle of centre C
passing through O (equation
) is at distance
of O.
The Kampyle can also be found as the rolling curve
of the linear
conchoidal motion, and as the base of the Kappa
motion (see this page).
| next curve | previous curve | 2D curves | 3D curves | surfaces | fractals | polyhedra |
© Robert FERRÉOL 2017