Catenary

CATENARY OR FUNICULAR CURVE

Curve studied by Leibniz, Jean Bernoulli and Huygens in 1691.
In its Latin form catenaria, the name is due to Huygens.
Other names: funicular/sail curve.
One can also find the name catenoid for affine catenary curves. This must not be confused with the catenoid surface.
See also: www.mathouriste.eu/Catenaire/Catenaire_Chainette.html

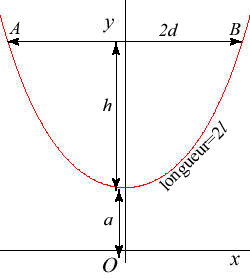
The relation between the length 2l, the sagitta h and the width 2d
is given by: , which results, by eliminating , in: i.e. .
Differential equation : .
Cartesian equation: .
The axis Ox is its base, the axis Oy is its symmetry axis.
Cartesian parametrization: ,
or , with .
Curvilinear algebra: .
Radius of curvature: (equal to the normal: -> Ribaucour's curve).
Intrinsic equation 1: (special case of an alysoid).
Intrinsic equation 2: (see the notations).
Transcendental curve.

The catenary is the shape taken by an inextensible, homogeneous, infinitely thin, flexible, massive wire hanging from two points, placed in a uniform gravitational field. Galileo posed the problem and observed that the curve followed by the wire was approximately an arc of
parabola, the approximation improves when you extend the wire. These are Leibniz, Jean Bernoulli and Huygens who found, independently in 1691, the right equations.

With the notations of the opposite figure ( = tension of the wire at M, = mass per unit length of the wire), we write that the sum of the forces at M is zero: .
This simplifies to, and, after integrating, .
We derive from this that which is the intrinsic differential equation 2 given above (see the notations).

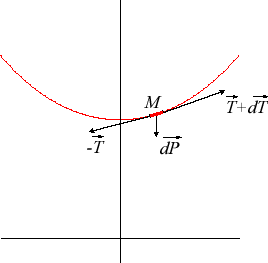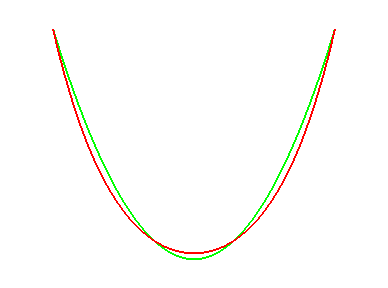
Let us learn how to distinguish catenaries from parabolas:

for an equal length, parabolas are "sharper".

See the curve of the suspension bridge, as well as the elastic catenary, that link the parabola and the catenary.
Like the suspended wire, a vault composed of edge-to-edge stones holding in place by their own weight also takes the shape of an upside-down catenary (property called "of the Poinsot vault"- proof Brocard part. comp. p 189):

The "Gateway Arch" in St Louis, Missouri has the shape of a catenary
Casa Mila rooftop, by the architect Gaudi in Barcelona.
Parking lot in Lyon
Dirigible hangar in Ecausseville

For surfaces of revolution having the same property, see dome of Bouguer.
On the contrary, the arches of bridges have a parabolic profile (upside-down suspension bridge...)

The Garabit viaduct has a parabolic arch (and not a circular one, as it is written on postcards)

The catenary also is the profile of a rectangular sail attached to two horizontal bars, swollen by a wind blowing perpendicularly to these bars, when the proper weight of the sail is neglected in comparison to the force of the wind, hence the name "sail curve" given by Jacques Bernoulli.

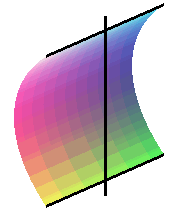
The arc of a curve of length l joining two given points A and B and for which the gravity centre is the farthest from the line (AB) (i.e. for which the potential energy is the lowest) is the arc of a catenary of length l joining A to B and that is symmetrical with respect to the perpendicular bisector of [AB] (but, if we want the maximal area delimited by a curve and [AB], the solution is half a circle).

The arc of a curve joining two given points A and B and for which the rotation around a line (D) coplanar with (AB) generates the surface of minimal area is the arc of a catenary of base (D) passing through A and B (see catenoid).

The catenary is still related to the parabola in the sense that it also is a parabolic roulette of Delaunay: the locus of the focus of a parabola rolling without slipping on a straight line.

The catenary is the only curve the radius of curvature of which equals the normal; the catenary is therefore the meridian line of the only surface of revolution with zero mean curvature: the catenoid; we deduce from this that the catenary is also a special case of Ribaucour curve.

The catenary is also the caustic by reflection of the exponential curve y = a e^x/a for rays parallel to Oy.

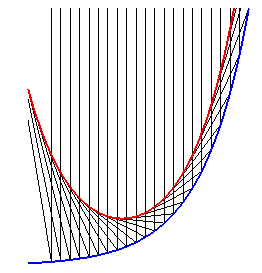
The evolute of the catenary is the curve with parametrization:

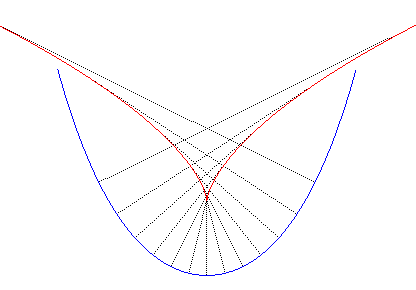The principal involute of the catenary is the tractrix the asymptote of which is the base of the catenary.

We derive from this that a point, fixed in the plane linked to a line rolling without slipping on the catenary, coinciding with the centre O of the latter when the line is tangent to its summit, describes the line Ox.

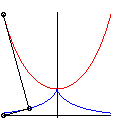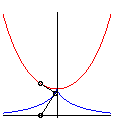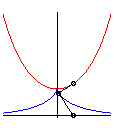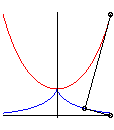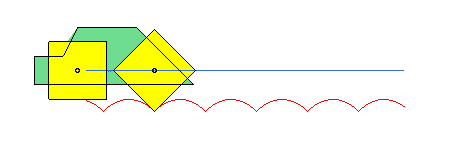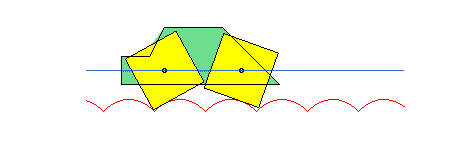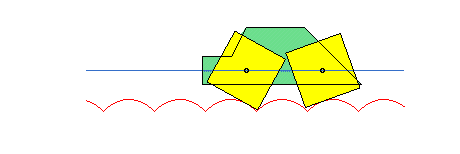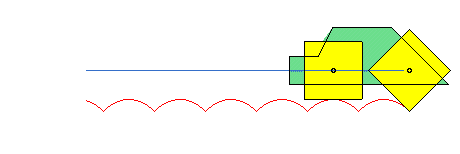
Thanks to this phenomenon, polygonal wheels can roll on arcs of catenaries while their centres move on a straight line.

For other funny wheels, see wheel-road couple.

The notion of catenary can be generalised to a wire placed on a surface, with the special case of the spherical catenary.

The wires of the funicular form a catenary when they are free, but the top of the funicular describes an elliptic arc....

Are these catenaries?

next curve previous curve 2D curves 3D curves surfaces fractals polyhedra