ELLIPSE
We advise the reader to read this page in parallel with that dedicated
to the hyperbola.
| next curve | previous curve | 2D curves | 3D curves | surfaces | fractals | polyhedra |
ELLIPSE
We advise the reader to read this page in parallel with that dedicated
to the hyperbola.
| From the Greek elleipein "miss".
Name given by Apollonius of Perga. See on this page by Alain Esculier the programs to draw the animations. |
 |
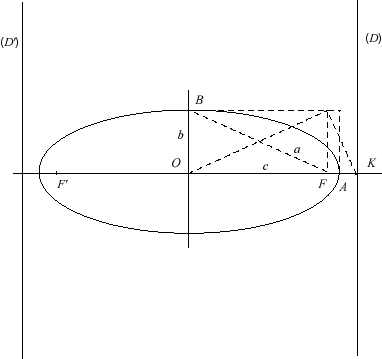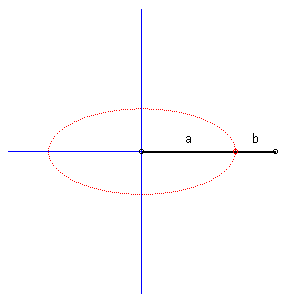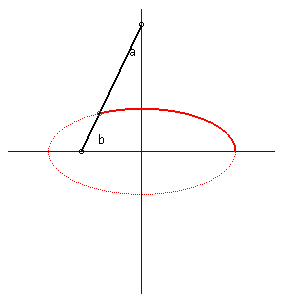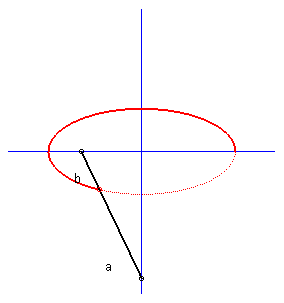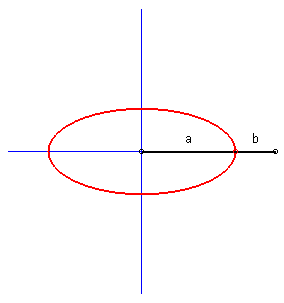
Reduced Cartesian equation:  .
a = semi-major axis ³ b = semi-minor axis > 0.  = linear
eccentricity.
= eccentricity, p = 
= semi-latus rectum,
 flattening
(with respect to the circle with radius a).
F(c, 0) et F'(-c, 0): foci of the ellipse. (D), (D'), lines with equation 
: directrices of the ellipse.
K: foot of the directrix on the axis Ox. d = FK =  . |
Cartesian parametrization:  .
Polar equation:  .
Pedal equation:  .
Curvilinear abscissa:  .
Radius of curvature: 
where N is the distance between M and the intersection point
of the normal with Ox.
Bifocal equation:  .
Polar equation (pole F, axis Ox):  .
Cartesian and polar equations in a frame with origin a summit and abscissa axis the focal axis: 
,  .
Length, given by an elliptic integral of the second kind:   . |
The ellipses are conics with eccentricity < 1.
They have been historically defined as section of the cone of revolution by a plane forming, with the axis of the cone, an angle greater than that formed by a generatrix and the axis:
ellipse = bounded intersection of a cone and a plane |
Application: the boundary of the trace of a light cone on a wall is elliptic, if it is bounded. |
but, more generally, any bounded section of a quadric by a plane is an ellipse.
The ellipse has numerous planar geometrical definitions:
1) Bifocal definition.
- the ellipse is the locus of the
points for which the sum of the distances to two fixed points F
and F' is constant (see the bifocal equation); hence the two following
constructions:
 |
 |
|
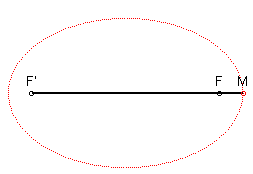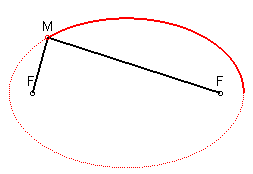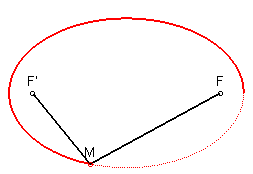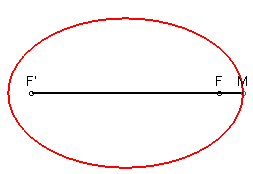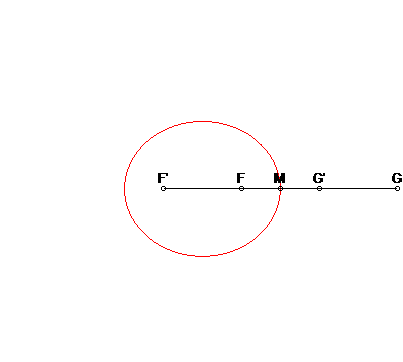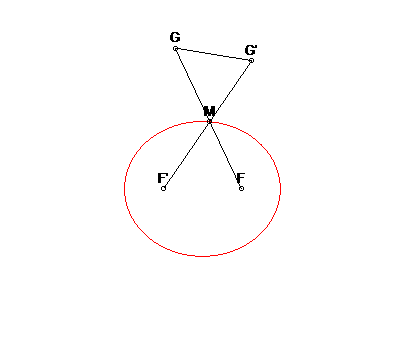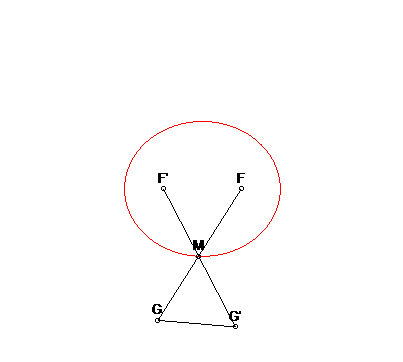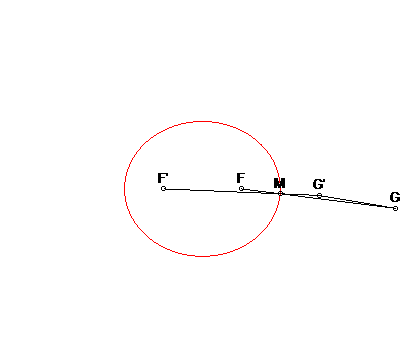See at inverse caustic, a generalisation of this construction. |
|
This definition can also be seen as "locus of the points for which the mean distance to two points is constant (equal to a)".
Application: 
Between the two pylons, this funicular describes an arc of an ellipse.
The construction of the foci and the directrix of the
ellipse defined as the section of a cone is given by the Dandelin theorem,
illustrated below:
 |
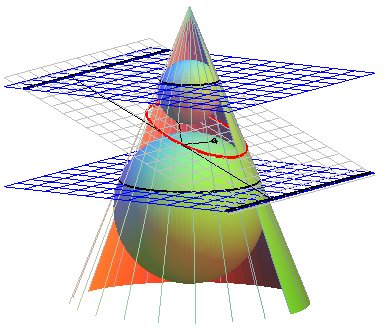The two spheres inscribed in the cone and tangent to the plane of the ellipse are tangent at its foci, and the planes of the contact circles cut the plane of the ellipse at the two directrices. |
For a planar generalisation of the property: ,
see the
Cayley ovals and
the Cartesian ovals; for a generalisation
to space, see the spherical
ellipse.
1 bis) The ellipse is the locus of a point M such that the tangent at M to this locus is the external bisector of the angle F'MF:
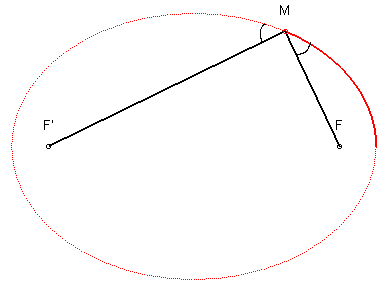
By the laws of reflection, any light ray (or acoustic wave) emitted by F is therefore reflected by the ellipse into a line passing by F'.
Applications:
|
In the Parisian subway, two people located at the two foci of the elliptic vault can talk to one another almost without being heard. Some rooms of the La Chaise Dieu abbey - to hear the lepers' confessions - have an odd property. Whispers in one place are clearly heard in another, but not in yet another... The same goes for the convent of the desert of the Lions near Mexico City (whispering gallery), at the Louvre museum, at the cathedral of Agrigento in Sicily, etc.... |
 |
 |
 On this elliptic billiard table, to put the ball in the hole after one bounce, one only has to aim for the other focus (which is not what the person in the picture is doing!) |
Elliptic speaker. |
| Other application: for any point M on the initial
ellipse, the symmetrical ellipse about the tangent at M is the ellipse
with foci G and G' seen above during the construction with
the three-bar.
Furthermore, this ellipse rolls without slipping on the first one. Note: the middle of the connecting rod [GG'], centre of the rolling ellipse, describes a Booth curve. |
 |
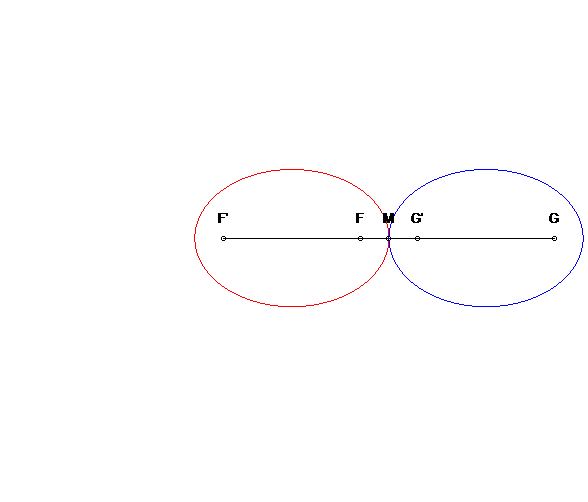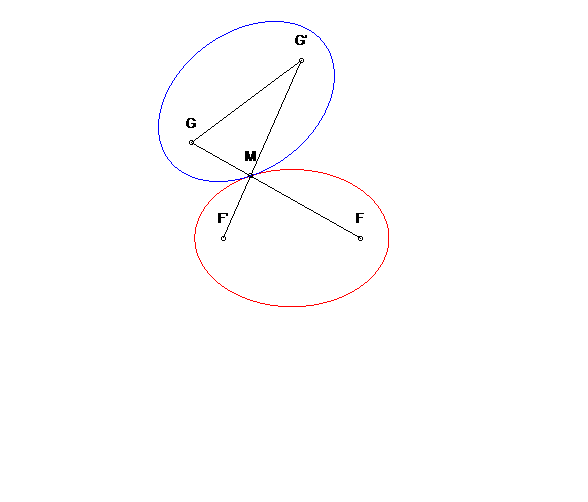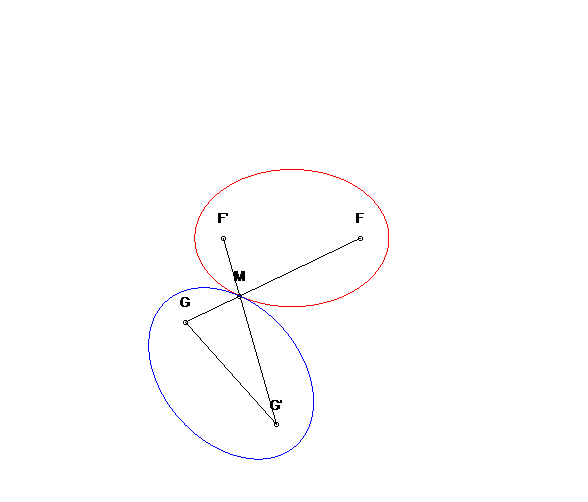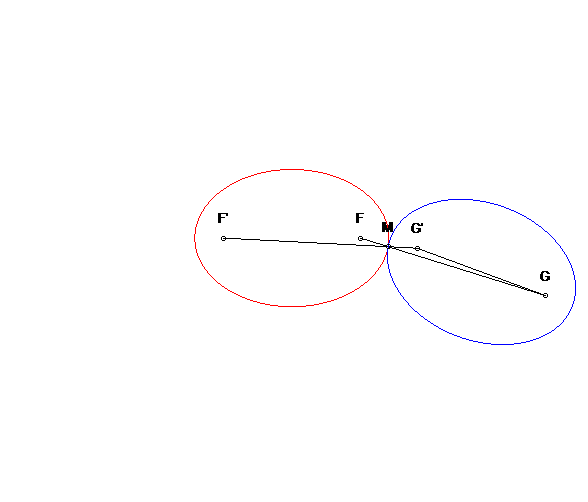| In the plane linked to F' and G', both the ellipses turn around F' and G' while always being tangent, and rolling without slipping: this property is called isotrépence of the ellipse with respect to a focus. |
 |
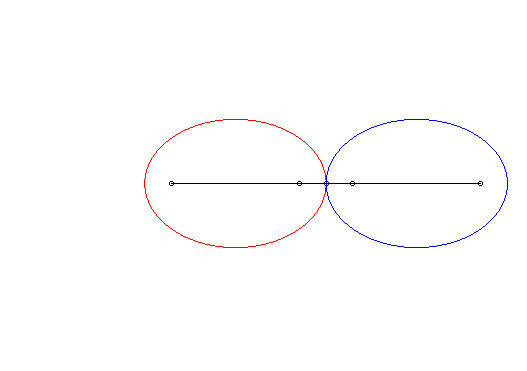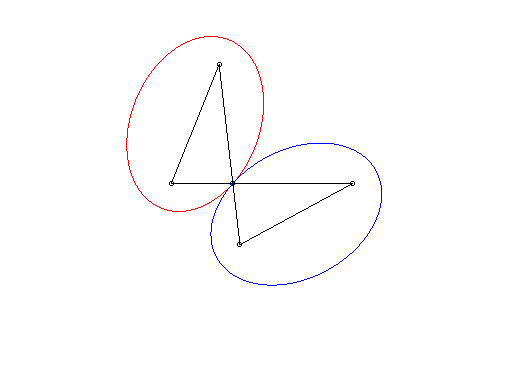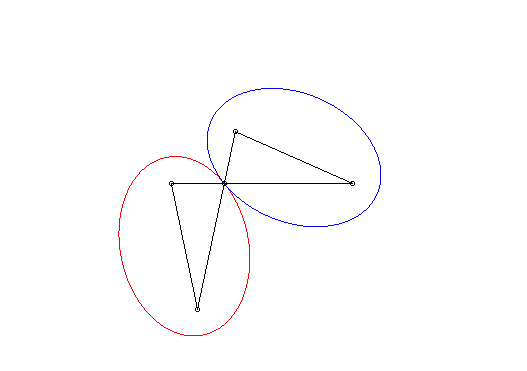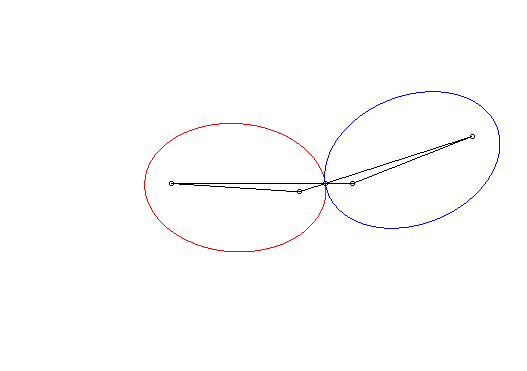2) Definition as an equidistance
curve between a point and a circle, in other words, as an isotel
of a circle.
The ellipse is the locus of the points at the same distance
from a circle (called the directrix circle, with centre one of the foci
F'
and radius 2a) and a point located inside the circle (which is the
other focus F); in other words, it is the locus of the centre of
a variable circle constrained to pass by F and be tangent to
C(F',
2a).
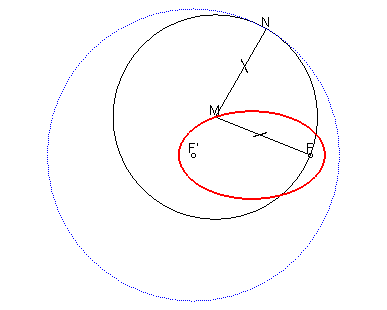
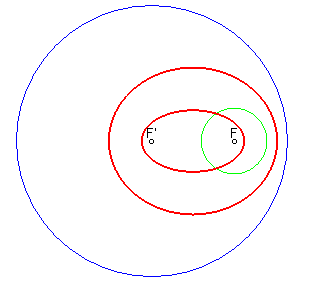
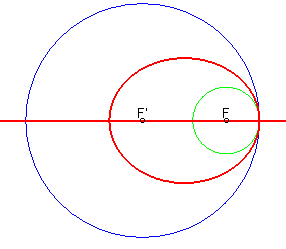
More generally, the equidistance
curves of two interior circles are reunions of ellipses:
| The red points are the "equidistant" points of the blue circle and the green one | ||
 |
 |
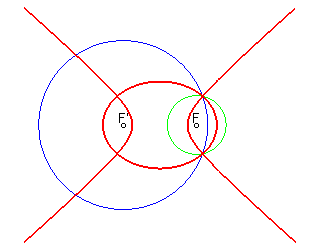
When the circles intersect, the equidistance curve is the reunion of an ellipse and a hyperbola. |
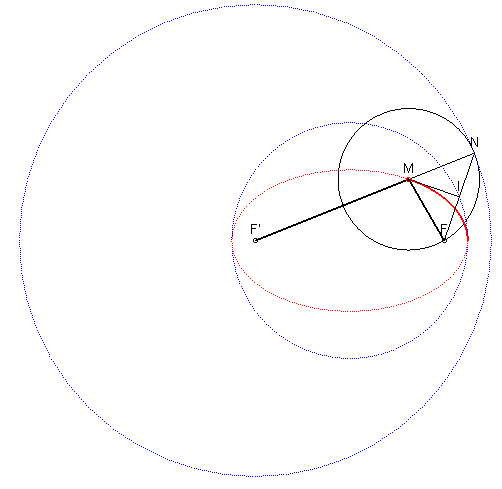
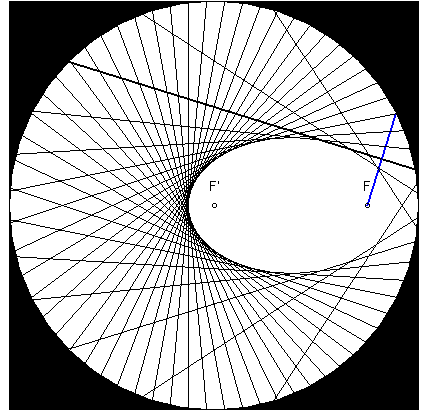
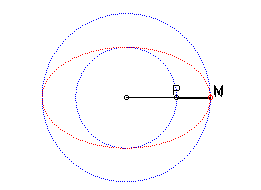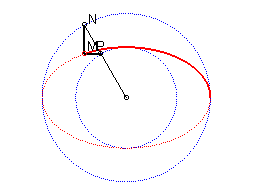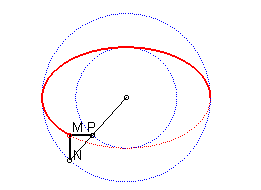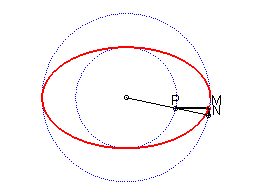
3) Tangential definition as the negative pedal of a circle.
The ellipse is the envelope of the line perpendicular
at I to the line (FI), when I describes the principal
circle C(O, a) (in other words, the negative
pedal of this circle with respect to F), or also the envelope
of the perpendicular bisector of the segment line [FN], when N
describes the directrix circle C(F', 2a) (which
is therefore the orthotomic
of the ellipse with respect to F).
 |
 |
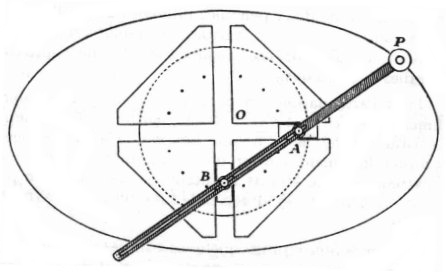
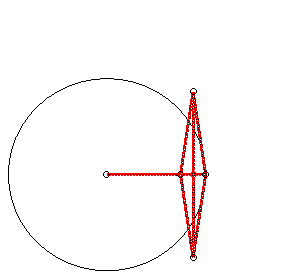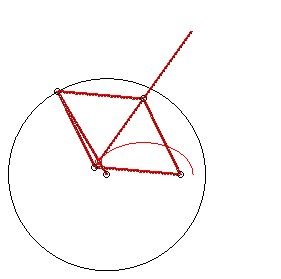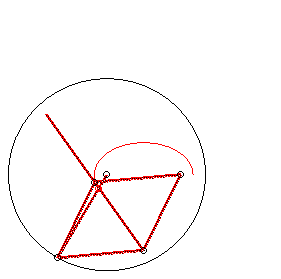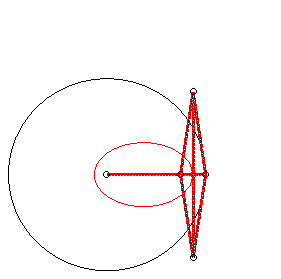
| This property is at the base of the construction of the
ellipse with a set-square for which the right angle describes a circle
and a side of which passes by a fixed point.
It is also at the base of the elegant construction by the articulated mechanism presented on the right. |
 |
 |
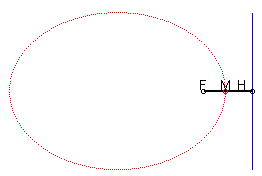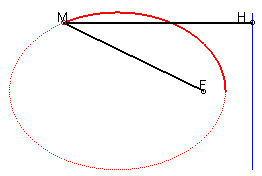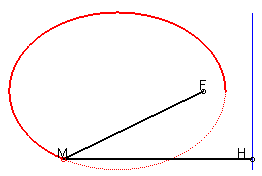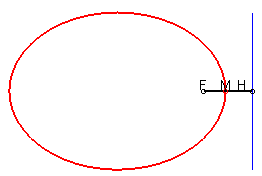
4) Definition by focus and directrix (not valid for the
circle).
The ellipse is the locus of a point M such that 
where H is the projection of M on the directrix (D),
with 0 < e < 1.
4 bis)
The ellipse is the locus of a point M such that
MF
+ eMH' = 2a where H' is the projection of M on
the directrix (D'), with 0 < e < 1.
| This means, when we interpret MF + eMH' as an
optical path length, that if the interior of the ellipse is composed of
a medium with refractive index n and the exterior's index is e.n,
then the incident rays parallel to the axis of the ellipse located on the
side of (D') will be refracted as rays passing, all of them, by
F.
In other words, the caustic by refraction of the ellipse for rays parallel to the axis reduces to the two foci. |
 |
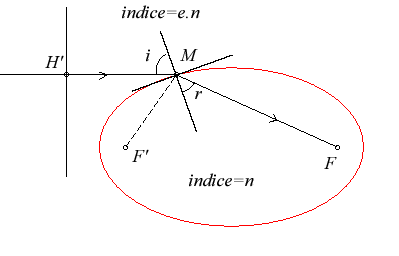
| This can be applied to the design of convergent lenses (the refractive index of the lens must be equal to 1/e): |  |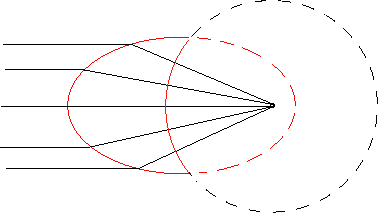
5) Definition by scaling in one direction of a circle.
The ellipse is the image by the scaling in one direction 
of the principal circle C(O, a) and also the image
by 
of the secondary circle C(O, b), hence the construction
called "by reduction of the ordinates", that shows that the ellipse is
image by the Newton transformation
of 2 concentric circles:
More generally, any affine function transforms a circle
into an ellipse; here are some applications:
 
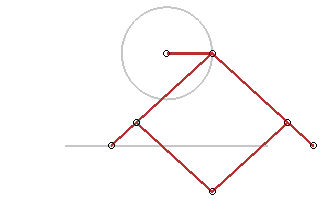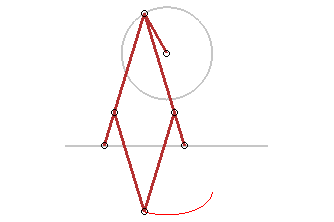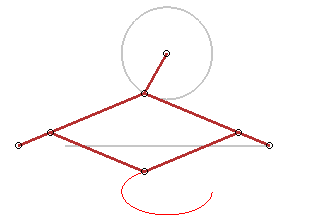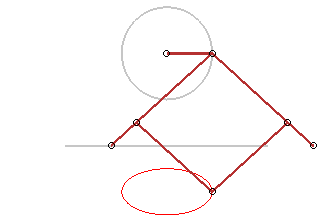
Ellipse-tracer of Delaunay |
 |
6) The ellipse is the locus of a point on a segment line
the ends of which move along non-parallel lines (and, by the way, of any
point linked to this segment line), hence the construction, called "with
a paper strip":
 
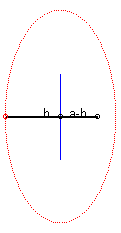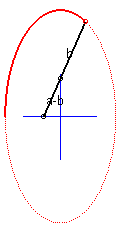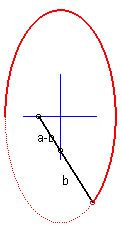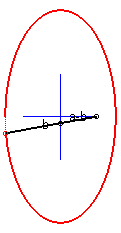
With this method, the same ellipse is obtained by two different ways. Note that the moving line envelopes an astroid. |
Ellipse-tracer of Archimedes (or Proclus), using this property |
Applications:
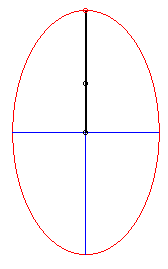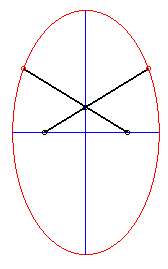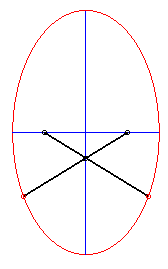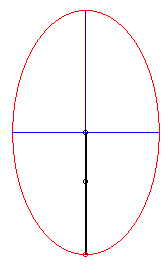
Scissors are opened by making the ends slide on a table; the points of the scissors describe an ellipse. |
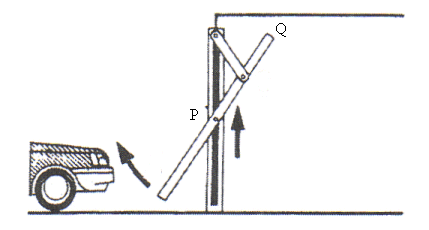
The points P and Q describe two perpendicular lines. |
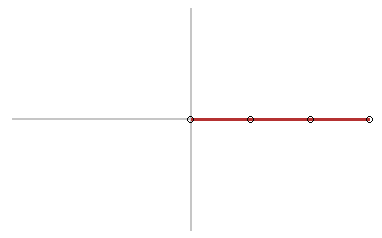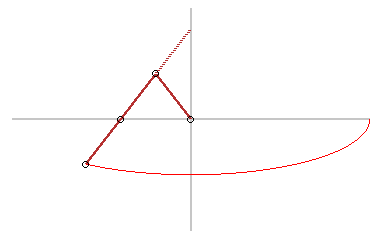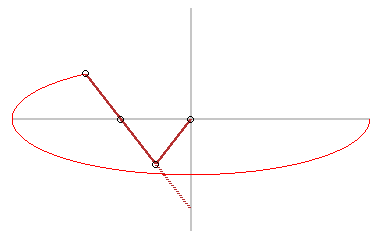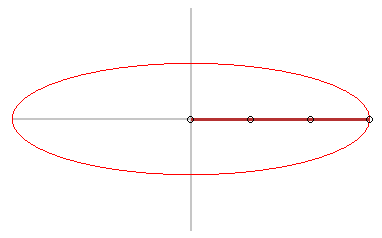
Hence another ellipse-tracer called "of Van Schooten" (that can also be considered as a slider-crank mechanism). |
For other curves obtained by articulated mechanisms, see three-bar curve.
7) Trochoidal definition.
|
The ellipse is a hypotrochoid, with a rolling circle with half radius compared to the base circle (composition of two circular motions with same angular speed in opposite directions). |
 |
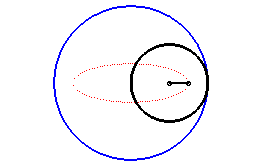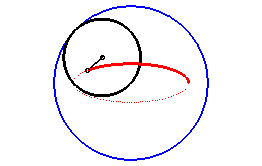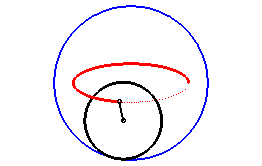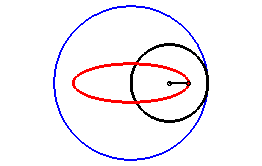| This definition is in fact related to the previous one, as shown in this figure ; the base of the movement of the plane linked to the segment line [A B] on the fixed plane is the blue circle and its rolling curve is the circle with diameter [A B], with a radius half as long. All the points in the moving plane describe ellipses (that are therefore the roulettes of this movement of a plane over a fixed plane). |
 |
8) Definition by conjugate diameters.
| Any line passing by the centre of the ellipse (diameter
of the ellipse) is an OBLIQUE axis of symmetry of the ellipse, the
direction of symmetry being given by the tangents at the intersection between
the axis and the ellipse. The diameter parallel to this direction is also
an oblique axis of symmetry, the direction being parallel to the first
diameter. The two diameters are said to be conjugate.
Conversely, the ellipse is the boundary of any convex set with oblique axes of symmetry in every direction. (BERGER th. 15.5.9). NB: in the parametrization |
 |
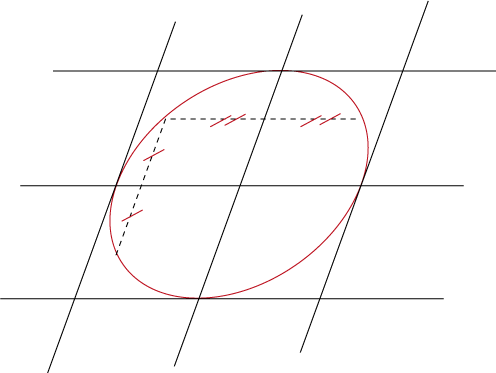9) The ellipse is a special case of Lissajous
curve.
_________________________________________________________________________________________________
|
The circles of curvature at the summits can be easily constructed, and allow for an approximate construction of the ellipse: |
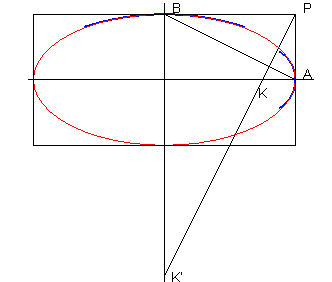
The blue circles can be easily drawn ((KK') perpendicular to (AB)) |
For the evolute of the ellipse, see tetracuspid;
the curves parallel to the ellipse are the toroids;
the negative pedal of the ellipse with respect to its centre is the Talbot
curve.
See also on this
page the movement of a plane over a fixed planed defined by an ellipse
constrained to pass by two fixed points.
Here is another mechanism, called "of Cavalieri", to trace
ellipses: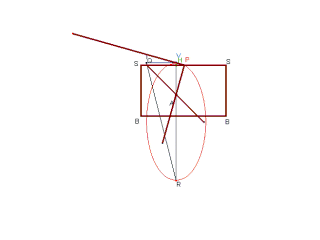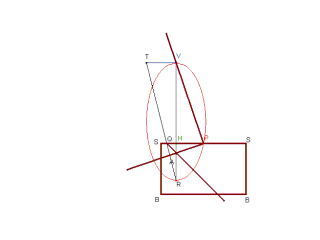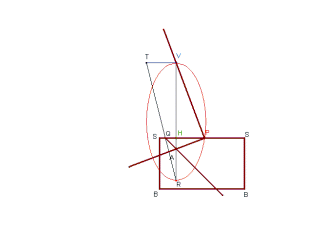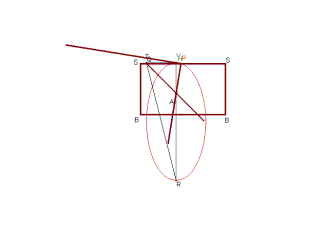 
.
 |
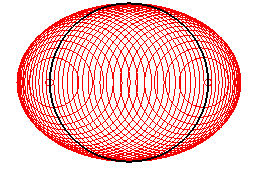The envelopes of the circles for which the ends of the
diameter parallel to a given direction lie on a circle is the ellipse the
foci of which are diametrically opposed on the circle and tangent to the
circle (flattening and eccentricity equal to 
). This property can be interpreted in 3 dimensions with the circles of
the ellipsoid, was
one of the three exercises Einstein had to solve for the Matura (Swiss
equivalent to the A-level exams), for which he received the maximal grade... |
Bridge in Brittany (photo by Mauricette Decamp) |
The Coliseum (that has an approximate elliptic shape, and not only in perspective!) |
| next curve | previous curve | 2D curves | 3D curves | surfaces | fractals | polyhedra |
© Robert FERRÉOL, Jacques MANDONNET, Alain ESCULIER 2017