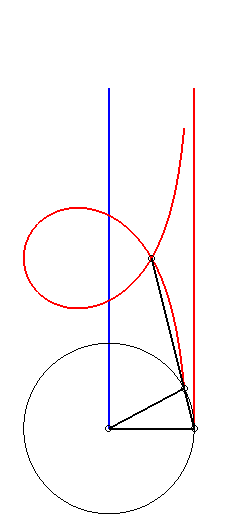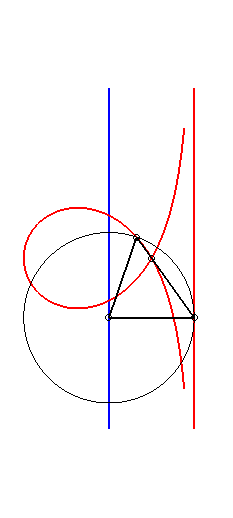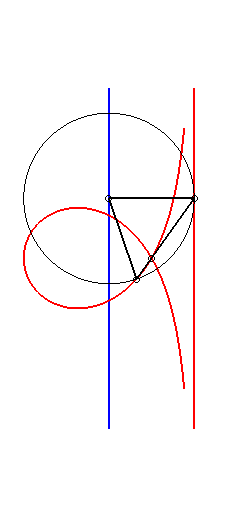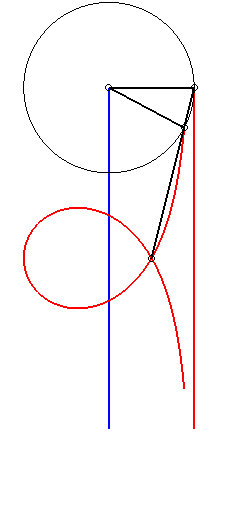
Cartesian equation: , i.e. . Rational quartic (singular point at O) located in the strip  with the line x = a as an asymptote if , and bounded otherwise. Polar equation: .

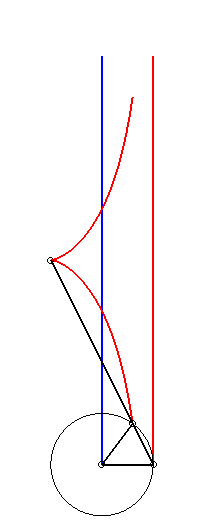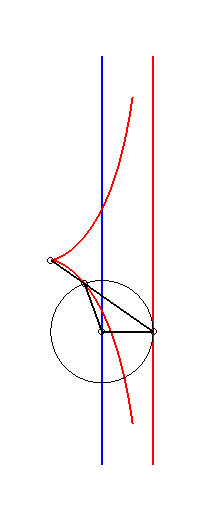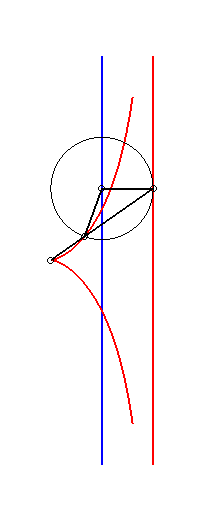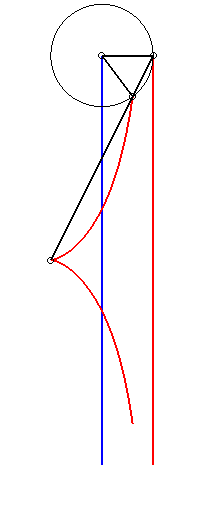
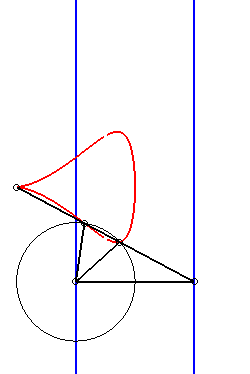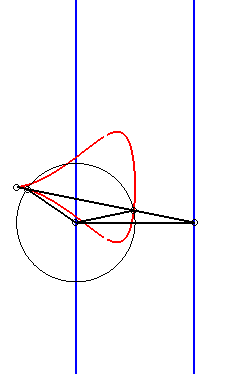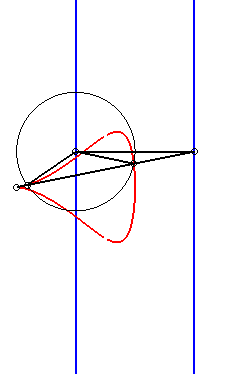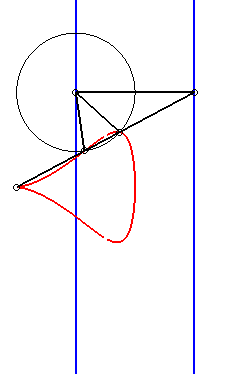
Given two points N and P with the same ordinate, respectively describing the lines x = a and x = b, the kieroid is the locus of the intersection points between the circle with centre P and radius c and the line (ON).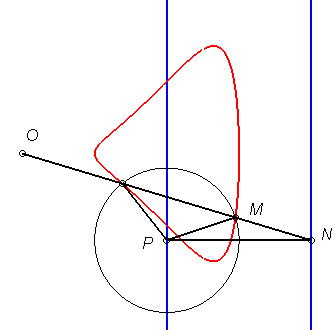

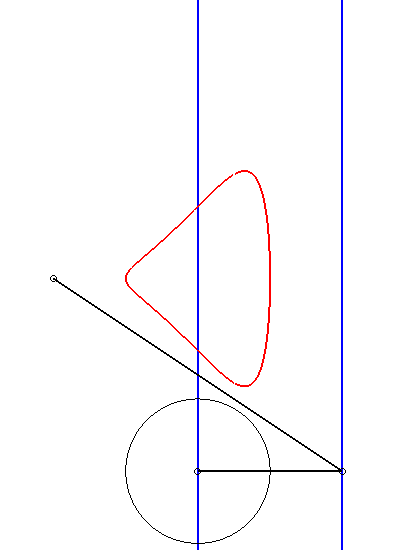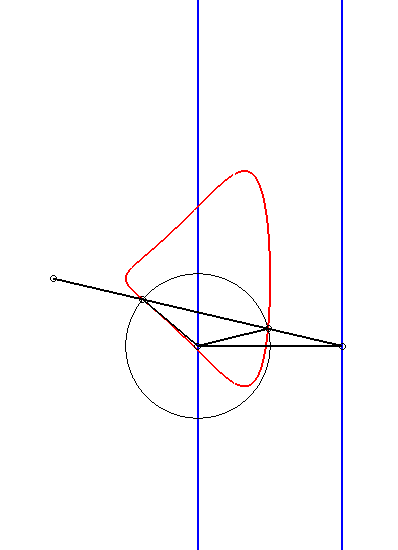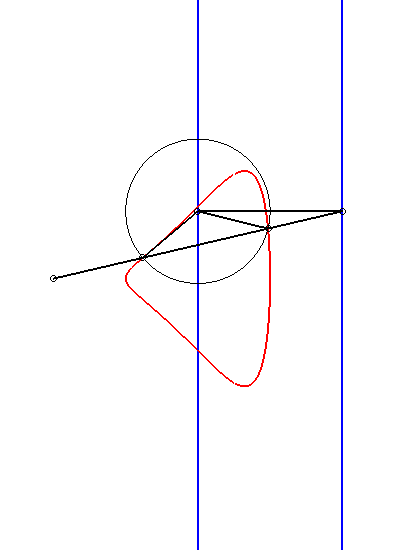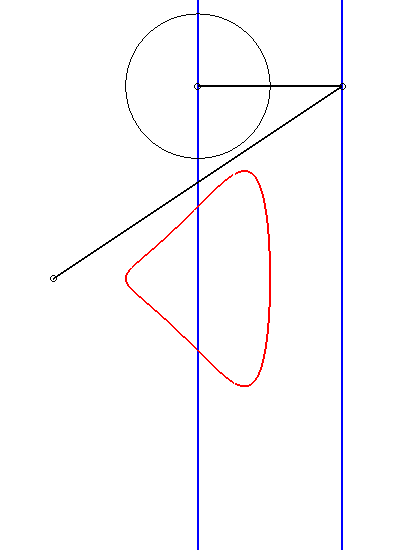When  (i.e. when the circle is tangent to the line x = a) , the kieroid can be decomposed into a right rational circular cubic and its asymptote x = a, and all the right rational circular cubics can be obtained this way, hence a new construction of these curves.

When a = b+c, the cubic can be written  or  with the special cases:
 

a = 2b = 2c: cissoid of Diocles	a = c, b = 0: right strophoid	2a = -2b = c: Mac-Laurin trisectrix

 

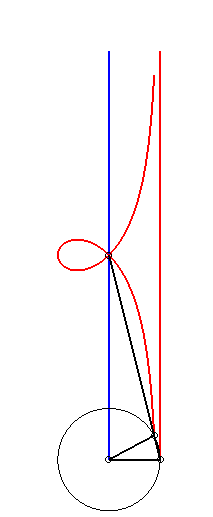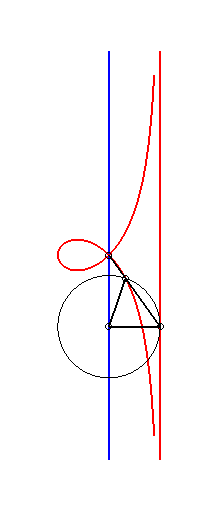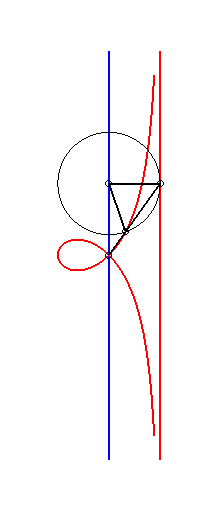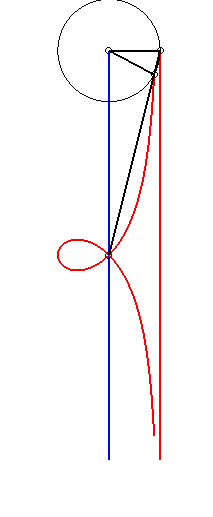When a = b - c, the cubic can be written or ; example: the visiera.

 

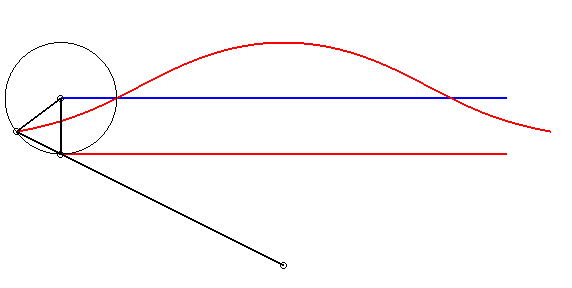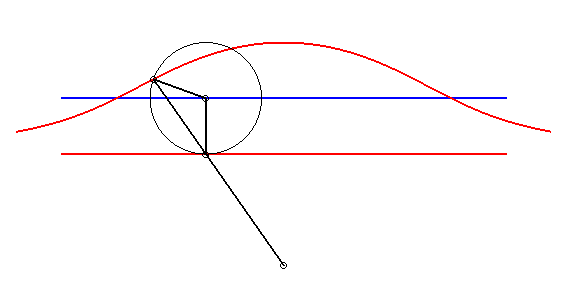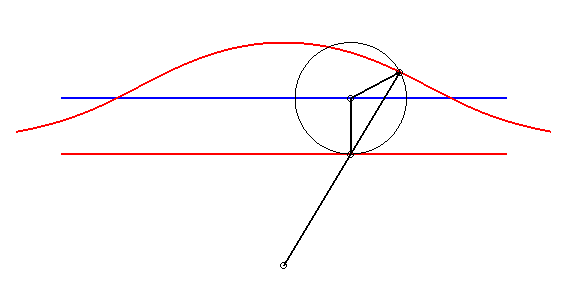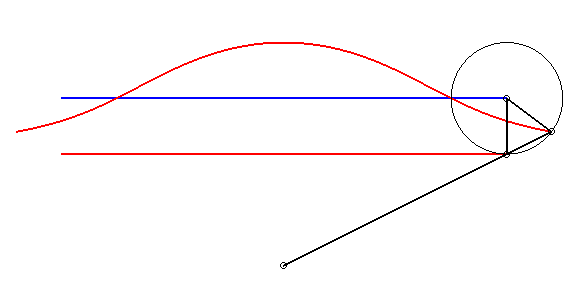When a = b, we get the conchoids of lines:  or .	When b = c, the kieroid has a cuspidal point at O; when, additionally, a >> 2b, the kieroid  gets closer to the piriform quartic

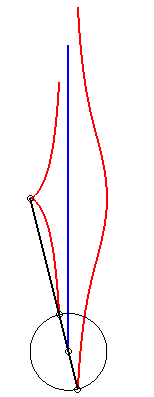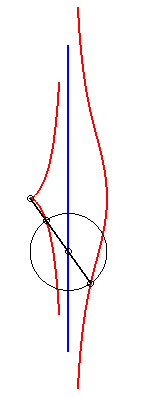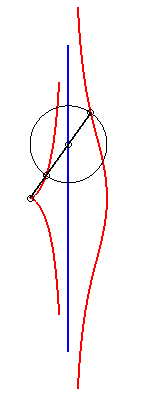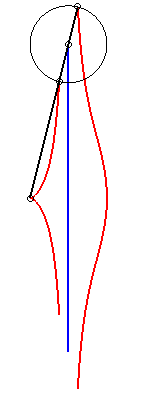Compare to the Rosillo curves.
 

next curve

previous curve

2D curves

3D curves

surfaces

fractals

polyhedra

© Robert FERRÉOL 2017