MACLAURIN TRISECTRIX
| next curve | previous curve | 2D curves | 3D curves | surfaces | fractals | polyhedra |
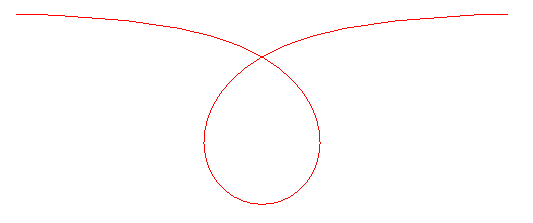
MACLAURIN TRISECTRIX

| Curve studied by Maclaurin in 1742.
Colin Maclaurin (1698-1746): Scottish mathematician. |
| Polar equation: Cartesian parametrization: Cartesian equation: Rational Cartesian parametrization: Crunodal right rational circular cubic. Polar equation in the frame (A(2a,0), Area of the loop = area between the curve and the asymptote = The tangents at O form angles of |
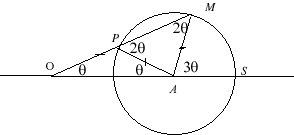
| Given two points O and S, the Maclaurin
trisectrix with vertex S (here S(3a, 0)) and double
point O is the locus of the points M such that OP
=PA = AM where A is defined by The angle SOM is the third of the angle SAM, hence the name of trisectrix: given the trisectrix and the points A and S, drawing a line passing by A gives the point M, so the angle SOM is the third of the angle SAM. If B is the point (4a, 0), it should be noted that the median (AI) of the triangle (ABM) is then also a trisectrix line (point A sees the segments [MI] and [IB] under double angles one of the other). |
 |
| Maclaurin imagined his curve from a trisection method
that was already known by the Greeks: draw a circle (C) with radius
R
and centre O passing by S and M; indicate on a stick
two points O and P at distance R from one another,
and
make O slide on the line (AS) and P slide on the circle
(C): when the stick passes by M, SOM trisects the
angle SAM.
Although the instruments used are the ruler and the compass, this is not a "ruler-and-compass construction" since the points O and P are not "constructed". |
 |
| The Maclaurin trisectrix is therefore also the locus of the intersection points between two lines, each in uniform rotation around a point, one of them going three times as fast as the other (see the generalisation at Maclaurin sectrix). |
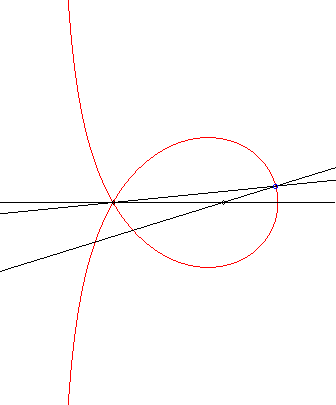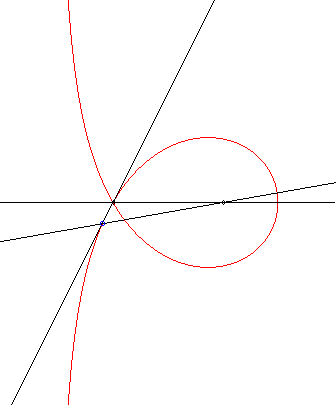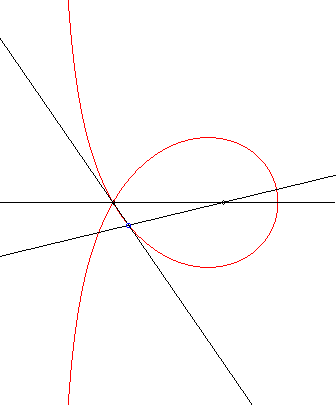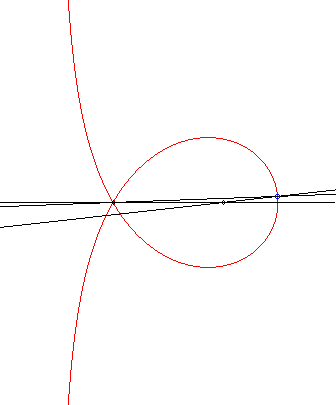 |
Like all rational
circular cubics, the Maclaurin trisectrix can also be defined as:
| - the cissoid with pole O of a circle passing by O and the symmetric image about O of the mediatrix of the radius passing by O (here, cissoid of the circle with centre W(2a,0) passing by O and of the line x = – a, with respect to O). |
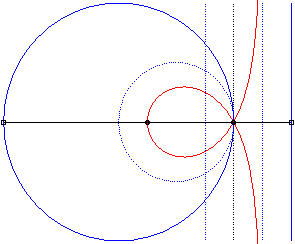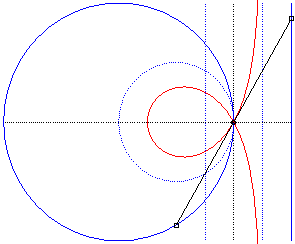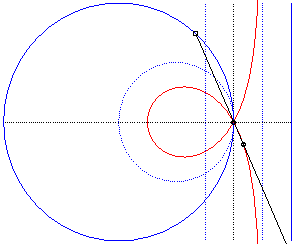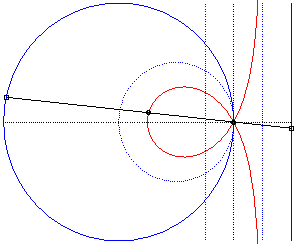 |
| - the pedal of
a parabola with respect to the symmetric image of the focus about the directrix
(here, the parabola with parameter 2a and vertex S, with
equation |
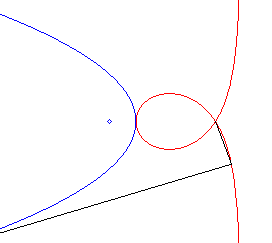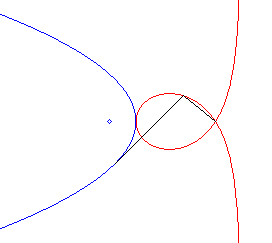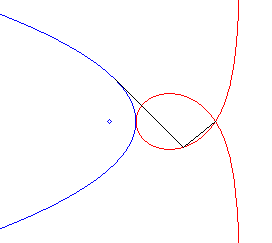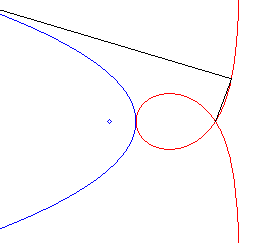 |
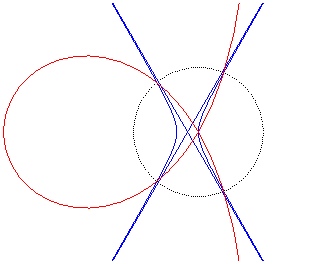
| - the inverse of a hyperbola with eccentricity 2 with respect to one of its vertices (here, the hyperbola with vertices O and (a/3, 0)) |
 |
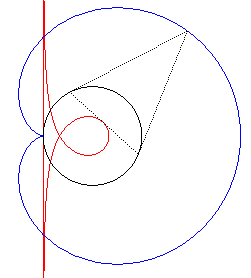
| Furthermore, the Maclaurin trisectrix is the polar of the cardioid with respect to the centre of its conchoidal circle: |
 |
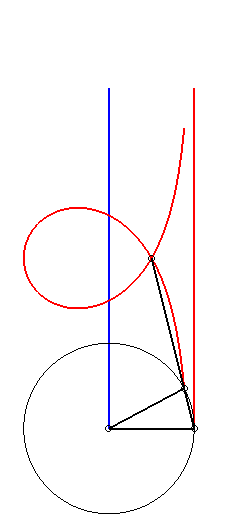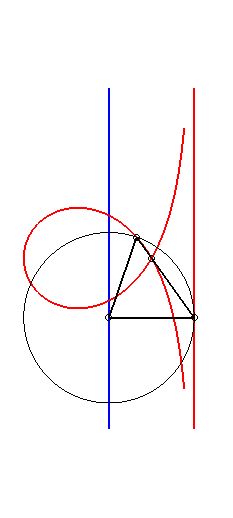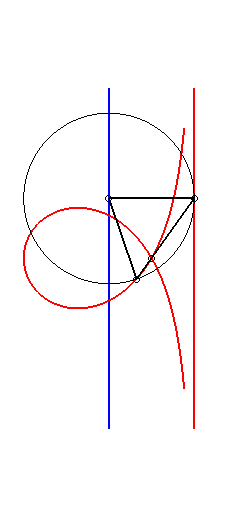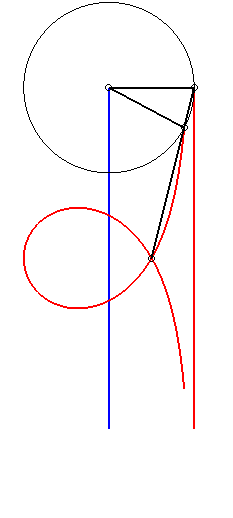
Moreover, like all right
rational circular cubics, the Maclaurin trisectrix can be constructed
|
|
|
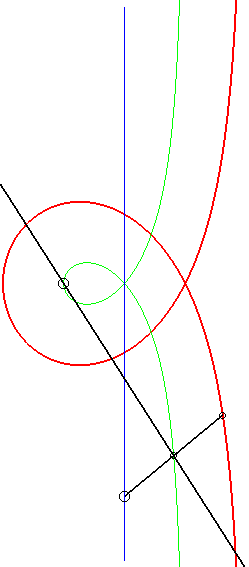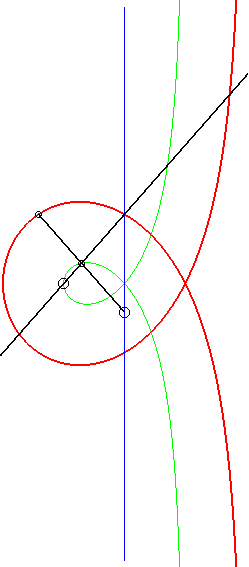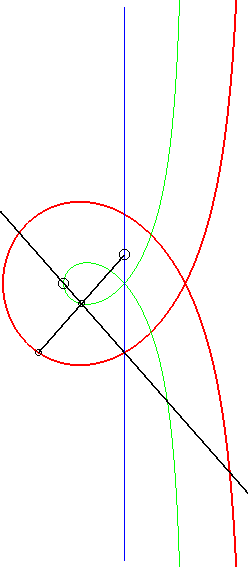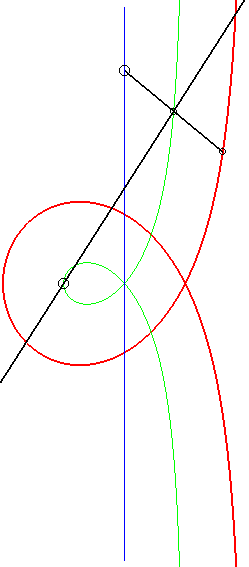 |
 |
The polar equation above shows that the Maclaurin trisectrix is also a special case of epispiral.
The Cartesian
folium is none other than a scaled Maclaurin trisectrix.
| next curve | previous curve | 2D curves | 3D curves | surfaces | fractals | polyhedra |
© Robert FERRÉOL 2017