où
1) 
with positive g, C1
on ]a, b[,
2) 
concave on [a, b] .
| next curve | previous curve | 2D curves | 3D curves | surfaces | fractals | polyhedra |
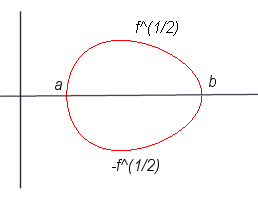
Oval
| From latin ovum "egg". |
An oval is a curve shaped like an egg.
In a general fashion, we can give the following definition:
curve of class C1
, boundary of a bounded convex subset of plane. Then, a closed curve of
class C1 all
the points of which have a positive curvature is an oval.
By restricting ourselves to curves having an axis of symmetry,
we can give the definition:
| Cartesian equation :
où
1) 2) |
|
Examples that fit this definition:
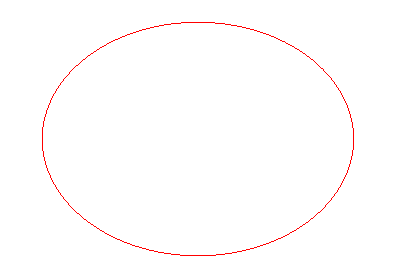
ellipse ( ,
k being the flattening of the ellipse), opposite with k = 3/4 |
 |
Tolstoy oval ( ),
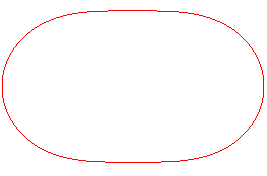
special case of Cassini oval |
 |
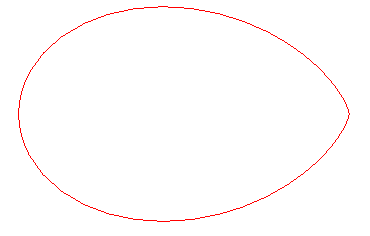
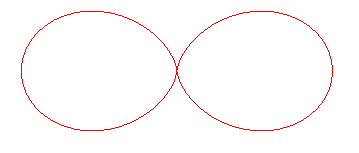
simple folium
( , a
= 0, b = 1) |
 |
half a dobble
egg ( ,
a = 0, b = 1) |
 |
oval of the cubical
hyperbola with an oval (
, 0 < a < b ), ci-contre avec a = 1, b
= 2, k = 3/4 |
 |
Granville
egg ( ,
0 < a < b) , ci-contre avec a = 4, b =
6, k = 4 |
 |
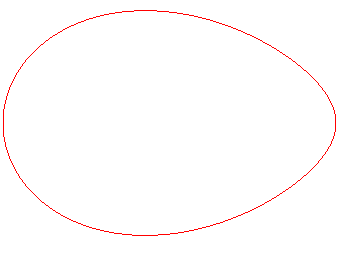
Rosillo curve
( ,
b = –
a, a < c < d ), ci-contre avec a = 1,
c
=
2, d = 3 |
 |
See also the Cartesian ovals, the Ehrhart eggs, the curves of the slider-crank mechanism, the right folia, the ovoïds obtained by rotation of an oval.
For a list of egg-like curves: www.mathematische-basteleien.de/eggcurves.htm
| next curve | pervious curve | 2D curves | 3D curves | surfaces | fractals | polyhedra |
© Robert FERRÉOL 2024