Other name: generalized helicoid. Alain Esculier's website.

 

For a spine curve  with current point  and curvilinear abscissa s, parametrization of the rotoid with generatrix :  where  and , the angle y is the angle of torsion of the spine curve (see the notations). h is the reduced shift of the rotoid and 2ph its shift.

The word rotoid refers to any surface generated by a regular screw of a curve (the generatrix) around a fixed curve (the "spine curve, or "bore" of the rotoid).
The intersection between the rotoid and a tube with same spine curve is a union of solenoids with reduced shift h.
When h is positive, the rotoid is said to be right-handed, and left-handed otherwise.

When the spine curve is linear, we get the helicoids.

When the generatrix is a line, we get the ruled rotoids, with similar definitions as those of the ruled helicoids.

The Möbius surface is a closed normal ruled rotoid with a circle as its spine curve.

When the center of a regular polygon moves along a curve, perpendicularly to it, with a regular torsion motion, the sides of the polygon trace a surface that we will call "rotoidal prism". Each "face" of this "prism" is a portion of open normal ruled rotoid.
For the case of a linear spine curve, see at ruled helicoid.

For the case of a circular spine curve, a (convex) polygon with n sides, and a torsion of k n-ths of a turn for one revolution, we get a surface composed of d = GCD(n, k) "faces" (after a revolution, the side #x of the polygon connects with the side #x+k modulo n). Moreover, there are also d "edges", which form a toroidal link of type (k, n): each component turns n/d times around the axis, and winds k/d times around the torus.
In particular, if n and k are coprime, the rotoidal prism has only one "face" and only one edge.

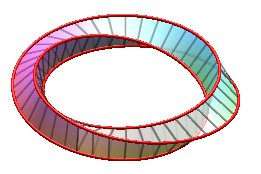
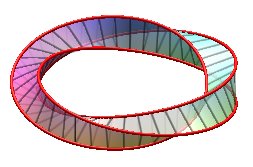
Examples for n = 3:
 

k = 1: one face, one untied edge	k = 2: one face, one edge tied as a trefoil knot	k = 3: three faces (fake Möbius strips with 2 half-twists), three edges (Villarceau circles of the torus, fake Borromean rings)	k = 4: one face, one edge, toroidal knot of the type (4,3), prime knot with 8 crossings.

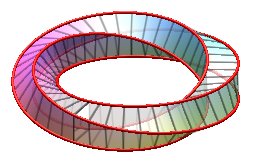
Examples for n = 4:
 

k = 1: one face, one untied edge	k = 2: two faces, two untied edges, forming a Salomon knot	k = 3: one face, on edge, toroidal knot of type (3,4), prime knot with 8 crossings.	k = 4: 4 faces (fake Möbius strips with 4 half-twists), 4 edges (Villarceau circles)
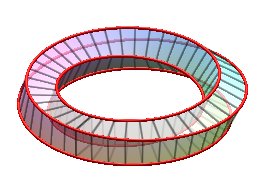
Was named monohedron by Jean-Pierre Petit

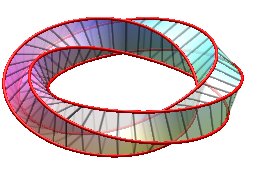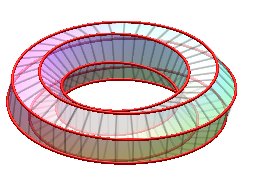
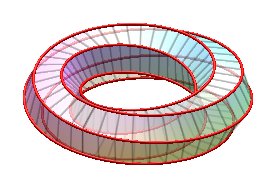
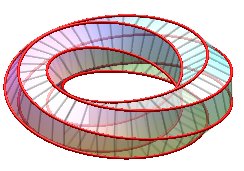
Examples for n = 6:
 

k = 1: one face, one untied edge	k = 2: two faces, two untied edges	k = 3: three faces, three edges, toroidal link of type (3,6).	k = 4: two faces, two untied edges, toroidal link of type (4,6).

 
 

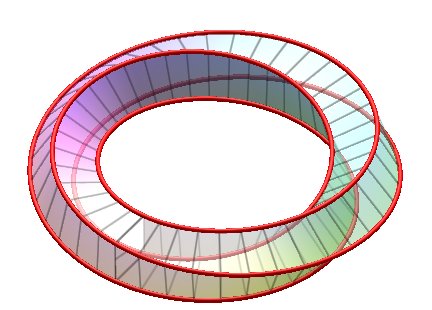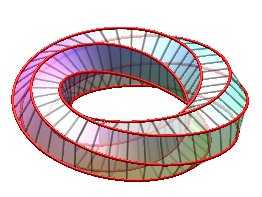
Opposite, a view of the case n = 4, k = 2; if the tracing square is contracted into a segment line, (in other words, if we stick two edges together), we get a Möbius strip. Therefore, this solid can also be seen as a Möbius strip cut in thick cardboard, the length of the strip being equal to the width of the cardboard.

 

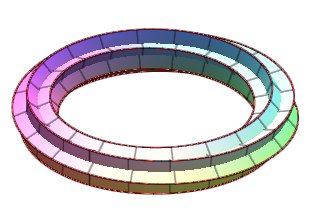
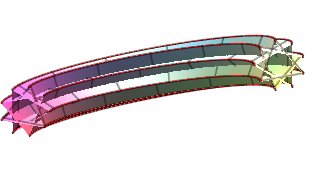
Generalization to the case where k is a rational number p/q. The linear section of the resulting surface is composed of q polygons with n sides, i.e. a polygram with symbol {qn/q}. Opposite, the case n = 3, p =1, q =2.

Game: find the values of n and k in the sculptures below:
 

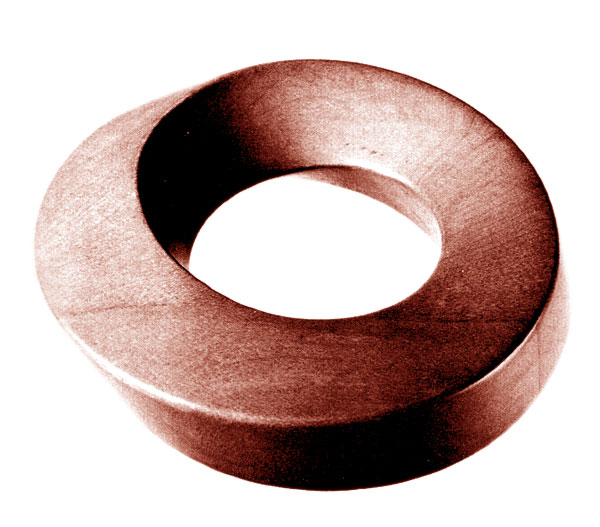	Work photographed in the Mentoring Museum of Tours	Work by Jean-Daniel Huyghe	Work by Christophe Chini

 

next surface

previous surface

2D curves

3D curves

surfaces

fractals

polyhedra

© Robert FERRÉOL  2017