
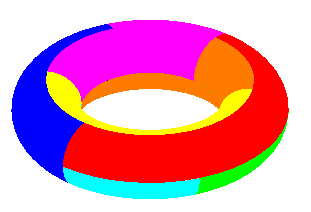
Torus obtained by the Maple instruction: tubeplot([cos(t),0,0],t=0..2*Pi,radius=2+sin(t)), close to a sphere with a tunnel
| next surface | previous surface | 2D curves | 3D curves | surfaces | fractals | polyhedra |
(TOPOLOGICAL) TORUS
| As a topological notion, the torus, or sphere with one handle, refers to any topological space homeomorphic to the Cartesian product of a circle by itself: |

Torus obtained by the Maple instruction: tubeplot([cos(t),0,0],t=0..2*Pi,radius=2+sin(t)), close to a sphere with a tunnel |
Characterization: orientable connected compact surface of genus 1 (or of Euler characteristic zero). Its mean Gaussian curvature is therefore equal to zero.
|
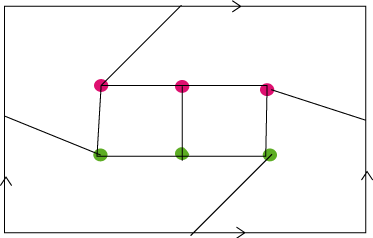
The torus is one of the 3 surfaces obtained by identifying, or in concrete terms, sewing together, the opposite sides of a square: |
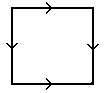
We sew the opposite sides in the same direction: we get a torus. |
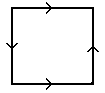 |
 We sew the opposite sides in opposite directions: we get a projective plane. |
| The chromatic number of the torus (minimum number of colors needed to color the countries of a map traced on the torus so that two countries with a common border have different colors) is equal to the maximum number of countries of a map for which all the countries have, 2 by 2, a common border, and is equal to 7.
To get a map of the torus with 7 countries, each touching the 6 others, we can start from a hexagonal pavement for which the hexagons are colored with 7 colors, the 6 hexagons that surround a given hexagon being of different colors. The parallelogram traced with the arrows that indicate the identifications gives a map of the torus with 7 adjacent countries.
|
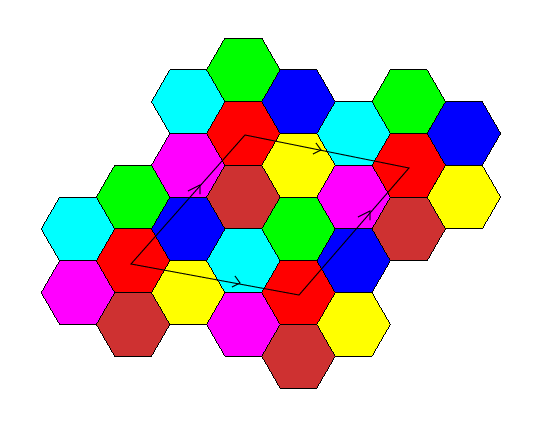 |
| Opposite, an example of a map with 7 countries traced on a "true" torus, each country touching the 6 others.
The Szillassi polyhedron achieves the prowess of providing a polyhedral version of this map. |
 |
| The three utilities problem has a solution on the torus, whereas it cannot be solved in the plane. In other words, the bipartite graph The same holds for the complete graph with 5 vertices The Csazar polyhedron achieves the prowess of providing a polyhedral version of this graph. |
 |
See also the sine surface and the octahemioctahedron which provide immersions of the torus.
This torus can be generalized into the n-dimensional torus.
| next surface | previous surface | 2D curves | 3D curves | surfaces | fractals | polyhedra |
© Robert FERRÉOL 2017